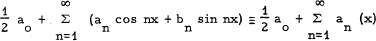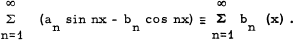Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Vinogradova, I. A.
and
Skvortsov, V. A.
1973.
Generalized Fourier integrals and series.
Journal of Soviet Mathematics,
Vol. 1,
Issue. 6,
p.
677.
Sklyarenko, V. A.
1980.
Limits of indeterminacy of the (C, k)-means of a trigonometric series and the cross Hk-integral.
Mathematical Notes of the Academy of Sciences of the USSR,
Vol. 28,
Issue. 3,
p.
645.
Lee, Cheng-Ming
1981.
On Integrals and Summable Trigonometric Series.
Canadian Mathematical Bulletin,
Vol. 24,
Issue. 4,
p.
433.