Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Anderson, D. F.
Dobbs, D. E.
and
Fontana, M.
1990.
Characterizing Kronecker function rings.
ANNALI DELL UNIVERSITA DI FERRARA,
Vol. 36,
Issue. 1,
p.
1.
Reinhart, Andreas
2014.
Commutative Algebra.
p.
307.
Izelgue, L.
Mimouni, A. Achour
and
Tamoussit, A.
2020.
On the Module Structure of the Integer-Valued Polynomial Rings.
Bulletin of the Malaysian Mathematical Sciences Society,
Vol. 43,
Issue. 3,
p.
2687.
El Baghdadi, S.
Izelgue, L.
and
Tamoussit, A.
2020.
Almost Krull domains and their rings of integer–valued polynomials.
Journal of Pure and Applied Algebra,
Vol. 224,
Issue. 6,
p.
106269.
Tamoussit, A.
2021.
A note on the Krull dimension of rings between D[X] and $$\mathrm {Int}(D)$$.
Bollettino dell'Unione Matematica Italiana,
Vol. 14,
Issue. 3,
p.
513.
Kim, Hwankoo
and
Tamoussit, Ali
2022.
Integral domains issued from associated primes.
Communications in Algebra,
Vol. 50,
Issue. 2,
p.
538.
Chems-Eddin, Mohamed Mahmoud
and
Tamoussit, Ali
2023.
Various properties of a general class of integer-valued polynomials.
Beiträge zur Algebra und Geometrie / Contributions to Algebra and Geometry,
Vol. 64,
Issue. 1,
p.
81.
Tamoussit, Ali
2023.
Algebraic, Number Theoretic, and Topological Aspects of Ring Theory.
p.
443.
Houston, Evan
and
Zafrullah, Muhammad
2023.
Well-behaved prime t-ideals and almost Krull domains.
Communications in Algebra,
Vol. 51,
Issue. 1,
p.
276.
Chang, Gyu Whan
Kim, Hwankoo
and
Tamoussit, Ali
2025.
Associated prime dimensions and
t
-dimensions in integral domains
.
Communications in Algebra,
Vol. 53,
Issue. 11,
p.
4778.

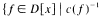 and let
and let 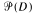 denote the set of prime ideals of
denote the set of prime ideals of 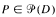 then Huckaba and Papick have asked whether
then Huckaba and Papick have asked whether 