No CrossRef data available.
Published online by Cambridge University Press: 20 April 2023
We show that a sufficiently general hypersurface of degree d in  $\mathbb {P}^n$ admits a toric Gröbner degeneration after linear change of coordinates if and only if
$\mathbb {P}^n$ admits a toric Gröbner degeneration after linear change of coordinates if and only if 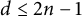 $d\leq 2n-1$.
$d\leq 2n-1$.
N.I. was supported by an NSERC Discovery Grant. O.L. was supported by an NSERC undergraduate student research award.