Published online by Cambridge University Press: 20 November 2018
Our main result is that many triangles of Baumslag–Solitar groups collapse to finite groups, generalizing a famous example of Hirsch and other examples due to several authors. A triangle of Baumslag–Solitar groups means a group with three generators, cyclically ordered, with each generator conjugating some power of the previous one to another power. There are six parameters, occurring in pairs, and we show that the triangle fails to be developable whenever one of the parameters divides its partner, except for a few special cases. Furthermore, under fairly general conditions, the group turns out to be finite and solvable of derived length 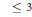 $\le \,3$. We obtain a lot of information about finite quotients, even when we cannot determine developability.
$\le \,3$. We obtain a lot of information about finite quotients, even when we cannot determine developability.