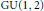Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Vollaard, Inken
and
Wedhorn, Torsten
2011.
The supersingular locus of the Shimura variety of GU(1,n−1) II.
Inventiones mathematicae,
Vol. 184,
Issue. 3,
p.
591.
Kudla, Stephen
and
Rapoport, Michael
2011.
Special cycles on unitary Shimura varieties I. Unramified local theory.
Inventiones mathematicae,
Vol. 184,
Issue. 3,
p.
629.
Vandenbergen, Nicolas
2013.
On the Global Structure of Special Cycles on Unitary Shimura Varieties.
Canadian Journal of Mathematics,
Vol. 65,
Issue. 5,
p.
1125.
Rapoport, Michael
Terstiege, Ulrich
and
Zhang, Wei
2013.
On the arithmetic fundamental lemma in the minuscule case.
Compositio Mathematica,
Vol. 149,
Issue. 10,
p.
1631.
Rapoport, Michael
Terstiege, Ulrich
and
Wilson, Sean
2014.
The supersingular locus of the Shimura variety for $$\hbox {GU}(1, n-1)$$ GU ( 1 , n - 1 ) over a ramified prime.
Mathematische Zeitschrift,
Vol. 276,
Issue. 3-4,
p.
1165.
Shen, Xu
2014.
Cell decomposition of some unitary group Rapoport–Zink spaces.
Mathematische Annalen,
Vol. 360,
Issue. 3-4,
p.
825.
Howard, Benjamin
and
Pappas, Georgios
2014.
On the supersingular locus of the GU(2,2) Shimura variety.
Algebra & Number Theory,
Vol. 8,
Issue. 7,
p.
1659.
de Shalit, Ehud
and
Goren, Eyal Z.
2016.
A theta operator on Picard modular forms modulo an inert prime.
Research in the Mathematical Sciences,
Vol. 3,
Issue. 1,
Li, Chao
and
Zhu, Yihang
2017.
Remarks on the arithmetic fundamental lemma.
Algebra & Number Theory,
Vol. 11,
Issue. 10,
p.
2425.
de Shalit, Ehud
and
Goren, Eyal Z.
2017.
Supersingular curves on Picard modular surfaces modulo an inert prime.
Journal of Number Theory,
Vol. 171,
Issue. ,
p.
391.
Rapoport, M.
Smithling, B.
and
Zhang, W.
2017.
On the arithmetic transfer conjecture for exotic smooth formal moduli spaces.
Duke Mathematical Journal,
Vol. 166,
Issue. 12,
Howard, Benjamin
and
Pappas, Georgios
2017.
Rapoport–Zink spaces for spinor groups.
Compositio Mathematica,
Vol. 153,
Issue. 5,
p.
1050.
Chen, Miaofen
and
Viehmann, Eva
2017.
Affine Deligne-Lusztig varieties and the action of 𝐽.
Journal of Algebraic Geometry,
Vol. 27,
Issue. 2,
p.
273.
de Shalit, Ehud
and
Goren, Eyal Z.
2018.
Geometry, Algebra, Number Theory, and Their Information Technology Applications.
Vol. 251,
Issue. ,
p.
81.
Rapoport, M.
Smithling, B.
and
Zhang, W.
2018.
Regular formal moduli spaces and arithmetic transfer conjectures.
Mathematische Annalen,
Vol. 370,
Issue. 3-4,
p.
1079.
Görtz, Ulrich
He, Xuhua
and
Nie, Sian
2019.
Fully Hodge–Newton Decomposable Shimura Varieties.
Peking Mathematical Journal,
Vol. 2,
Issue. 2,
p.
99.
Li, Wanlin
Mantovan, Elena
Pries, Rachel
and
Tang, Yunqing
2019.
Newton polygons arising from special families of cyclic covers of the projective line.
Research in Number Theory,
Vol. 5,
Issue. 1,
HE, XUHUA
LI, CHAO
and
ZHU, YIHANG
2019.
FINE DELIGNE–LUSZTIG VARIETIES AND ARITHMETIC FUNDAMENTAL LEMMAS.
Forum of Mathematics, Sigma,
Vol. 7,
Issue. ,
Wang, Haining
2020.
On the Bruhat–Tits stratification of a quaternionic unitary Rapoport–Zink space.
Mathematische Annalen,
Vol. 376,
Issue. 3-4,
p.
1107.
Li, Chao
and
Zhang, Wei
2021.
Kudla–Rapoport cycles and derivatives of local densities.
Journal of the American Mathematical Society,
Vol. 35,
Issue. 3,
p.
705.