Article contents
Spaces of knotted circles and exotic smooth structures
Published online by Cambridge University Press: 24 August 2020
Abstract
Suppose that 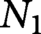 $N_1$ and
$N_1$ and  $N_2$ are closed smooth manifolds of dimension n that are homeomorphic. We prove that the spaces of smooth knots,
$N_2$ are closed smooth manifolds of dimension n that are homeomorphic. We prove that the spaces of smooth knots, 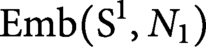 $ \operatorname {\mathrm {Emb}}(\mathrm {S}^1, N_1)$ and
$ \operatorname {\mathrm {Emb}}(\mathrm {S}^1, N_1)$ and  $ \operatorname {\mathrm {Emb}}(\mathrm {S}^1, N_2),$ have the same homotopy
$ \operatorname {\mathrm {Emb}}(\mathrm {S}^1, N_2),$ have the same homotopy 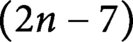 $(2n-7)$-type. In the four-dimensional case, this means that the spaces of smooth knots in homeomorphic
$(2n-7)$-type. In the four-dimensional case, this means that the spaces of smooth knots in homeomorphic 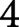 $4$-manifolds have sets
$4$-manifolds have sets 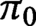 $\pi _0$ of components that are in bijection, and the corresponding path components have the same fundamental groups
$\pi _0$ of components that are in bijection, and the corresponding path components have the same fundamental groups 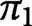 $\pi _1$. The result about
$\pi _1$. The result about  $\pi _0$ is well-known and elementary, but the result about
$\pi _0$ is well-known and elementary, but the result about 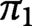 $\pi _1$ appears to be new. The result gives a negative partial answer to a question of Oleg Viro. Our proof uses the Goodwillie–Weiss embedding tower. We give a new model for the quadratic stage of the Goodwillie–Weiss tower, and prove that the homotopy type of the quadratic approximation of the space of knots in N does not depend on the smooth structure on N. Our results also give a lower bound on
$\pi _1$ appears to be new. The result gives a negative partial answer to a question of Oleg Viro. Our proof uses the Goodwillie–Weiss embedding tower. We give a new model for the quadratic stage of the Goodwillie–Weiss tower, and prove that the homotopy type of the quadratic approximation of the space of knots in N does not depend on the smooth structure on N. Our results also give a lower bound on  $\pi _2 \operatorname {\mathrm {Emb}}(\mathrm {S}^1, N)$. We use our model to show that for every choice of basepoint, each of the homotopy groups,
$\pi _2 \operatorname {\mathrm {Emb}}(\mathrm {S}^1, N)$. We use our model to show that for every choice of basepoint, each of the homotopy groups, 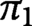 $\pi _1$ and
$\pi _1$ and 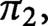 $\pi _2,$ of
$\pi _2,$ of 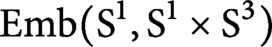 $ \operatorname {\mathrm {Emb}}(\mathrm {S}^1, \mathrm {S}^1\times \mathrm {S}^3)$ contains an infinitely generated free abelian group.
$ \operatorname {\mathrm {Emb}}(\mathrm {S}^1, \mathrm {S}^1\times \mathrm {S}^3)$ contains an infinitely generated free abelian group.
MSC classification
Information
- Type
- Article
- Information
- Creative Commons
- This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
- Copyright
- © Canadian Mathematical Society 2020
Footnotes
The authors thank the Isaac Newton Institute for Mathematical Sciences, Cambridge, for support and hospitality during the program “Homotopy harnessing higher structures” where work on this paper was undertaken. This work was supported by EPSRC grant no EP/K032208/1. G. A. was supported by the Swedish Research Council, grant number 2016-05440.
References
- 3
- Cited by


