Article contents
Perfect Orderings on Finite Rank Bratteli Diagrams
Published online by Cambridge University Press: 20 November 2018
Abstract
Given a Bratteli diagram  $B$ , we study the set
$B$ , we study the set 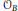 ${{\mathcal{O}}_{B}}$ of all possible orderings on
${{\mathcal{O}}_{B}}$ of all possible orderings on  $B$ and its subset
$B$ and its subset 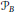 ${{\mathcal{P}}_{B}}$ consisting of perfect orderings that produce Bratteli–Vershik topological dynamical systems (Vershik maps). We give necessary and sufficient conditions for the ordering
${{\mathcal{P}}_{B}}$ consisting of perfect orderings that produce Bratteli–Vershik topological dynamical systems (Vershik maps). We give necessary and sufficient conditions for the ordering 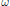 $\omega $ to be perfect. On the other hand, a wide class of non-simple Bratteli diagrams that do not admit Vershik maps is explicitly described. In the case of finite rank Bratteli diagrams, we show that the existence of perfect orderings with a prescribed number of extreme paths constrains significantly the values of the entries of the incidence matrices and the structure of the diagram
$\omega $ to be perfect. On the other hand, a wide class of non-simple Bratteli diagrams that do not admit Vershik maps is explicitly described. In the case of finite rank Bratteli diagrams, we show that the existence of perfect orderings with a prescribed number of extreme paths constrains significantly the values of the entries of the incidence matrices and the structure of the diagram  $B$ . Our proofs are based on the new notions of skeletons and associated graphs, defined and studied in the paper. For a Bratteli diagram
$B$ . Our proofs are based on the new notions of skeletons and associated graphs, defined and studied in the paper. For a Bratteli diagram  $B$ of rank
$B$ of rank  $k$ , we endow the set
$k$ , we endow the set 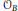 ${{\mathcal{O}}_{B}}$ with product measure
${{\mathcal{O}}_{B}}$ with product measure  $\mu $ and prove that there is some
$\mu $ and prove that there is some 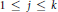 $1\,\le \,j\,\le \,k$ such that
$1\,\le \,j\,\le \,k$ such that  $\mu $ -almost all orderings on
$\mu $ -almost all orderings on  $B$ have
$B$ have 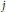 $j$ maximal and
$j$ maximal and 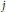 $j$ minimal paths. If
$j$ minimal paths. If 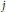 $j$ is strictly greater than the number of minimal components that
$j$ is strictly greater than the number of minimal components that  $B$ has, then
$B$ has, then  $\mu $ -almost all orderings are imperfect.
$\mu $ -almost all orderings are imperfect.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2014
References
- 8
- Cited by

