Article contents
On Pointwise Estimates of Positive Definite Functions With Given Support
Published online by Cambridge University Press: 20 November 2018
Abstract
The following problem has been suggested by Paul Turán. Let  $\Omega $ be a symmetric convex body in the Euclidean space
$\Omega $ be a symmetric convex body in the Euclidean space 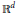 ${{\mathbb{R}}^{d}}$ or in the torus
${{\mathbb{R}}^{d}}$ or in the torus 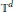 ${{\mathbb{T}}^{d}}$ . Then, what is the largest possible value of the integral of positive definite functions that are supported in
${{\mathbb{T}}^{d}}$ . Then, what is the largest possible value of the integral of positive definite functions that are supported in  $\Omega $ and normalized with the value 1 at the origin? From this, Arestov, Berdysheva and Berens arrived at the analogous pointwise extremal problem for intervals in
$\Omega $ and normalized with the value 1 at the origin? From this, Arestov, Berdysheva and Berens arrived at the analogous pointwise extremal problem for intervals in 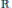 $\mathbb{R}$ . That is, under the same conditions and normalizations, the supremum of possible function values at
$\mathbb{R}$ . That is, under the same conditions and normalizations, the supremum of possible function values at  $z$ is to be found for any given point
$z$ is to be found for any given point 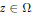 $z\,\in \,\Omega $ . However, it turns out that the problem for the real line has already been solved by Boas and Kac, who gave several proofs and also mentioned possible extensions to
$z\,\in \,\Omega $ . However, it turns out that the problem for the real line has already been solved by Boas and Kac, who gave several proofs and also mentioned possible extensions to 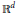 ${{\mathbb{R}}^{d}}$ and to non-convex domains as well.
${{\mathbb{R}}^{d}}$ and to non-convex domains as well.
Here we present another approach to the problem, giving the solution in 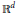 ${{\mathbb{R}}^{d}}$ and for several cases in
${{\mathbb{R}}^{d}}$ and for several cases in 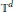 ${{\mathbb{T}}^{d}}$ . Actually, we elaborate on the fact that the problem is essentially one-dimensional and investigate non-convex open domains as well. We show that the extremal problems are equivalent to some more familiar ones concerning trigonometric polynomials, and thus find the extremal values for a few cases. An analysis of the relationship between the problem for
${{\mathbb{T}}^{d}}$ . Actually, we elaborate on the fact that the problem is essentially one-dimensional and investigate non-convex open domains as well. We show that the extremal problems are equivalent to some more familiar ones concerning trigonometric polynomials, and thus find the extremal values for a few cases. An analysis of the relationship between the problem for 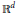 ${{\mathbb{R}}^{d}}$ and that for
${{\mathbb{R}}^{d}}$ and that for 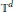 ${{\mathbb{T}}^{d}}$ is given, showing that the former case is just the limiting case of the latter. Thus the hierarchy of difficulty is established, so that extremal problems for trigonometric polynomials gain renewed recognition.
${{\mathbb{T}}^{d}}$ is given, showing that the former case is just the limiting case of the latter. Thus the hierarchy of difficulty is established, so that extremal problems for trigonometric polynomials gain renewed recognition.
Keywords
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2006
References
- 4
- Cited by

