Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Caprace, Pierre-Emmanuel
and
Rémy, Bertrand
2009.
Simplicity and superrigidity of twin building lattices.
Inventiones mathematicae,
Vol. 176,
Issue. 1,
p.
169.
Caprace, Pierre-Emmanuel
and
Rémy, Bertrand
2010.
Non-distortion of twin building lattices.
Geometriae Dedicata,
Vol. 147,
Issue. 1,
p.
397.
Caprace, Pierre-Emmanuel
and
Fujiwara, Koji
2010.
Rank-One Isometries of Buildings and Quasi-Morphisms of Kac–Moody Groups.
Geometric and Functional Analysis,
Vol. 19,
Issue. 5,
p.
1296.
CAPRACE, PIERRE-EMMANUEL
and
MONOD, NICOLAS
2011.
Decomposing locally compact groups into simple pieces.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 150,
Issue. 1,
p.
97.
Caprace, Pierre-Emmanuel
and
Monod, Nicolas
2012.
A lattice in more than two Kac-Moody groups is arithmetic.
Israel Journal of Mathematics,
Vol. 190,
Issue. 1,
p.
413.
Vavrichek, Diane M.
2012.
On the coarse-geometric detection of subgroups.
Geometriae Dedicata,
Vol. 156,
Issue. 1,
p.
93.
Baumgartner, Udo
Möller, Rögnvaldur G.
and
Willis, George A.
2012.
Hyperbolic groups have flat-rank at most 1.
Israel Journal of Mathematics,
Vol. 190,
Issue. 1,
p.
365.
Nakagawa, Yoshiyuki
Tamura, Makoto
and
Yamashita, Yasushi
2014.
Non-hyperbolic automatic groups and groups acting on CAT(0) cube complexes.
International Journal of Algebra and Computation,
Vol. 24,
Issue. 06,
p.
795.
Chao, Khek Lun Harold
2014.
CAT(0) spaces with boundary the join of two Cantor sets.
Algebraic & Geometric Topology,
Vol. 14,
Issue. 2,
p.
1107.
Lam, Thomas
and
Thomas, Anne
2015.
Infinite Reduced Words and the Tits Boundary of a Coxeter Group.
International Mathematics Research Notices,
Vol. 2015,
Issue. 17,
p.
7690.
Genevois, Anthony
2017.
Hyperbolic diagram groups are free.
Geometriae Dedicata,
Vol. 188,
Issue. 1,
p.
33.
Baumgartner, U.
Parkinson, J.
and
Ramagge, J.
2019.
Scale and tidy subgroups for Weyl-transitive automorphism groups of buildings.
Journal of Algebra,
Vol. 520,
Issue. ,
p.
460.
Genevois, Anthony
2021.
Algebraic characterisation of relatively hyperbolic special groups.
Israel Journal of Mathematics,
Vol. 241,
Issue. 1,
p.
301.
Kramer, Linus
2022.
Some remarks on proper actions, proper metric spaces, and buildings.
Advances in Geometry,
Vol. 22,
Issue. 4,
p.
541.
Genevois, Anthony
2023.
CAT(0) cube complexes with flat hyperplanes.
Proceedings of the American Mathematical Society,
Vol. 151,
Issue. 7,
p.
2749.
Davis, Michael W.
2024.
Infinite Group Actions on Polyhedra.
Vol. 77,
Issue. ,
p.
89.
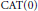 $\text{CAT}(0)$ space
$\text{CAT}(0)$ space 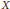 $X$ endowed with a geometric action of a group
$X$ endowed with a geometric action of a group 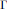 $\Gamma $ , it is known that if
$\Gamma $ , it is known that if 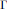 $\Gamma $ contains a free abelian group of rank
$\Gamma $ contains a free abelian group of rank  $n$ , then
$n$ , then 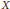 $X$ contains a geometric flat of dimension
$X$ contains a geometric flat of dimension  $n$ . We prove the converse of this statement in the special case where
$n$ . We prove the converse of this statement in the special case where 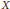 $X$ is a convex subcomplex of the
$X$ is a convex subcomplex of the 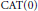 $\text{CAT}(0)$ realization of a Coxeter group
$\text{CAT}(0)$ realization of a Coxeter group  $W$ , and
$W$ , and 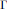 $\Gamma $ is a subgroup of
$\Gamma $ is a subgroup of  $W$ . In particular a convex cocompact subgroup of a Coxeter group is Gromov-hyperbolic if and only if it does not contain a free abelian group of rank 2. Our result also provides an explicit control on geometric flats in the
$W$ . In particular a convex cocompact subgroup of a Coxeter group is Gromov-hyperbolic if and only if it does not contain a free abelian group of rank 2. Our result also provides an explicit control on geometric flats in the 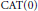 $\text{CAT}(0)$ realization of arbitrary Tits buildings.
$\text{CAT}(0)$ realization of arbitrary Tits buildings.
