Published online by Cambridge University Press: 20 November 2018
It is a well known fact that for rapidly increasing nkthe sequence 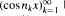 , behaves like a sequence of independent random variables; in particular
, behaves like a sequence of independent random variables; in particular 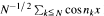 has a limiting Gaussian distribution as N → ∞. Under a certain critical speed
has a limiting Gaussian distribution as N → ∞. Under a certain critical speed 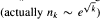 this result breaks down and
this result breaks down and 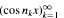 becomes strongly dependent. The purpose of this paper is to investigate the asymptotic behavior of normed sums
becomes strongly dependent. The purpose of this paper is to investigate the asymptotic behavior of normed sums 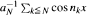 in the strongly dependent domain; specifically, we construct a large class of nongaussian limit distributions of such sums.
in the strongly dependent domain; specifically, we construct a large class of nongaussian limit distributions of such sums.