Article contents
New Simple Lattices in Products of Trees and their Projections
Published online by Cambridge University Press: 07 October 2019
Abstract
Let 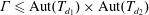 $\unicode[STIX]{x1D6E4}\leqslant \text{Aut}(T_{d_{1}})\times \text{Aut}(T_{d_{2}})$ be a group acting freely and transitively on the product of two regular trees of degree
$\unicode[STIX]{x1D6E4}\leqslant \text{Aut}(T_{d_{1}})\times \text{Aut}(T_{d_{2}})$ be a group acting freely and transitively on the product of two regular trees of degree 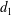 $d_{1}$ and
$d_{1}$ and  $d_{2}$. We develop an algorithm that computes the closure of the projection of
$d_{2}$. We develop an algorithm that computes the closure of the projection of  $\unicode[STIX]{x1D6E4}$ on
$\unicode[STIX]{x1D6E4}$ on  $\text{Aut}(T_{d_{t}})$ under the hypothesis that
$\text{Aut}(T_{d_{t}})$ under the hypothesis that 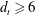 $d_{t}\geqslant 6$ is even and that the local action of
$d_{t}\geqslant 6$ is even and that the local action of 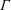 $\unicode[STIX]{x1D6E4}$ on
$\unicode[STIX]{x1D6E4}$ on 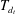 $T_{d_{t}}$ contains
$T_{d_{t}}$ contains 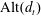 $\text{Alt}(d_{t})$. We show that if
$\text{Alt}(d_{t})$. We show that if  $\unicode[STIX]{x1D6E4}$ is torsion-free and
$\unicode[STIX]{x1D6E4}$ is torsion-free and 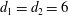 $d_{1}=d_{2}=6$, exactly seven closed subgroups of
$d_{1}=d_{2}=6$, exactly seven closed subgroups of  $\text{Aut}(T_{6})$ arise in this way. We also construct two new infinite families of virtually simple lattices in
$\text{Aut}(T_{6})$ arise in this way. We also construct two new infinite families of virtually simple lattices in 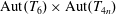 $\text{Aut}(T_{6})\times \text{Aut}(T_{4n})$ and in
$\text{Aut}(T_{6})\times \text{Aut}(T_{4n})$ and in  $\text{Aut}(T_{2n})\times \text{Aut}(T_{2n+1})$, respectively, for all
$\text{Aut}(T_{2n})\times \text{Aut}(T_{2n+1})$, respectively, for all 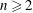 $n\geqslant 2$. In particular, we provide an explicit presentation of a torsion-free infinite simple group on 5 generators and 10 relations, that splits as an amalgamated free product of two copies of
$n\geqslant 2$. In particular, we provide an explicit presentation of a torsion-free infinite simple group on 5 generators and 10 relations, that splits as an amalgamated free product of two copies of 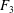 $F_{3}$ over
$F_{3}$ over 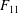 $F_{11}$. We include information arising from computer-assisted exhaustive searches of lattices in products of trees of small degrees. In an appendix by Pierre-Emmanuel Caprace, some of our results are used to show that abstract and relative commensurator groups of free groups are almost simple, providing partial answers to questions of Lubotzky and Lubotzky–Mozes–Zimmer.
$F_{11}$. We include information arising from computer-assisted exhaustive searches of lattices in products of trees of small degrees. In an appendix by Pierre-Emmanuel Caprace, some of our results are used to show that abstract and relative commensurator groups of free groups are almost simple, providing partial answers to questions of Lubotzky and Lubotzky–Mozes–Zimmer.
MSC classification
Information
- Type
- Article
- Information
- Copyright
- © Canadian Mathematical Society 2019
Footnotes
N. Radu is an F.R.S.-FNRS research fellow. P.-E. Caprace is an F.R.S.-FNRS senior research associate.
References
- 1
- Cited by


