Published online by Cambridge University Press: 20 November 2018
Let 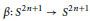 $\beta:\,{{S}^{2n+1}}\,\to \,{{S}^{2n+1}}$ be a minimal homeomorphism
$\beta:\,{{S}^{2n+1}}\,\to \,{{S}^{2n+1}}$ be a minimal homeomorphism  $\left( n\,\ge \,1 \right)$. We show that the crossed product
$\left( n\,\ge \,1 \right)$. We show that the crossed product 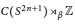 $C\left( {{S}^{2n+1}} \right)\,{{\rtimes }_{\beta}}\mathbb{Z}$ has rational tracial rank at most one. Let
$C\left( {{S}^{2n+1}} \right)\,{{\rtimes }_{\beta}}\mathbb{Z}$ has rational tracial rank at most one. Let 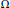 $\Omega $ be a connected, compact, metric space with finite covering dimension and with
$\Omega $ be a connected, compact, metric space with finite covering dimension and with 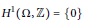 ${{H}^{1}}\left( \Omega ,\,\mathbb{Z} \right)\,=\,\left\{ 0 \right\}$. Suppose that
${{H}^{1}}\left( \Omega ,\,\mathbb{Z} \right)\,=\,\left\{ 0 \right\}$. Suppose that  ${{K}_{i}}\left( C\left( \Omega \right) \right)\,=\,\mathbb{Z}\,\oplus \,{{G}_{i}}$, where
${{K}_{i}}\left( C\left( \Omega \right) \right)\,=\,\mathbb{Z}\,\oplus \,{{G}_{i}}$, where 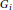 ${{G}_{i}}$ is a finite abelian group,
${{G}_{i}}$ is a finite abelian group,  $i\,=\,0,\,1$. Let
$i\,=\,0,\,1$. Let 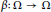 $\beta :\,\Omega \,\to \,\Omega $ be a minimal homeomorphism. We also show that
$\beta :\,\Omega \,\to \,\Omega $ be a minimal homeomorphism. We also show that 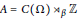 $A\,=\,C\left( \Omega \right)\,{{\rtimes }_{\beta}}\,\mathbb{Z}$ has rational tracial rank at most one and is classifiable. In particular, this applies to the minimal dynamical systems on odd dimensional real projective spaces. This is done by studying minimal homeomorphisms on
$A\,=\,C\left( \Omega \right)\,{{\rtimes }_{\beta}}\,\mathbb{Z}$ has rational tracial rank at most one and is classifiable. In particular, this applies to the minimal dynamical systems on odd dimensional real projective spaces. This is done by studying minimal homeomorphisms on  $X\,\times \,\Omega $, where
$X\,\times \,\Omega $, where 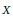 $X$ is the Cantor set.
$X$ is the Cantor set.