Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Balay, Meijke
Neutgens, Trent
Rosen, Nick
Wagner, Nathan
and
Zeytuncu, Yunus E.
2022.
$$L^p$$ regularity of Toeplitz operators on generalized Hartogs triangles.
European Journal of Mathematics,
Vol. 8,
Issue. 1,
p.
403.
Almughrabi, Rasha
2023.
Bergman Kernels of Two Dimensional Monomial Polyhedra.
Complex Analysis and Operator Theory,
Vol. 17,
Issue. 6,
Dall’Ara, G.
and
Monguzzi, A.
2023.
Nonabelian Ramified Coverings and $$L^p$$-boundedness of Bergman Projections in $${\mathbb {C}}^2$$.
The Journal of Geometric Analysis,
Vol. 33,
Issue. 2,
Chen, Liwei
Jin, Muzhi
and
Yuan, Yuan
2023.
Bergman Projection on the Symmetrized Bidisk.
The Journal of Geometric Analysis,
Vol. 33,
Issue. 7,
Ebenfelt, Peter
Xiao, Ming
and
Xu, Hang
2024.
Algebraicity of the Bergman kernel.
Mathematische Annalen,
Vol. 389,
Issue. 4,
p.
3417.
Jing, Yuanyuan
Li, Yi
Qin, Chuan
and
Wang, Mengjiao
2024.
Weak-Type Regularity of the Bergman Projection on n-Dimensional Hartogs Triangles.
Complex Analysis and Operator Theory,
Vol. 18,
Issue. 1,
Li, Yi
and
Wang, Mengjiao
2024.
Weak-type regularity for the Bergman projection over N-dimensional classical Hartogs triangles.
Journal of Inequalities and Applications,
Vol. 2024,
Issue. 1,
Huo, Zhenghui
and
Wick, Brett D.
2024.
$L^p$
regularity of the Bergman projection on the symmetrized polydisc.
Canadian Journal of Mathematics,
p.
1.
Chakrabarti, Debraj
and
Edholm, Luke D.
2024.
Projections onto L-Bergman spaces of Reinhardt domains.
Advances in Mathematics,
Vol. 451,
Issue. ,
p.
109790.
Chakrabarti, Debraj
Cinzori, Isaac
Gaidhane, Ishani
Gregory, Jonathan
and
Wright, Mary
2024.
Bergman kernels of monomial polyhedra.
Journal of Mathematical Analysis and Applications,
Vol. 531,
Issue. 1,
p.
127723.
Su, Guicong
Tang, Yanyan
and
Zhang, Shuo
2024.
The weighted Bergman kernels and projections on a class of unbounded Hartogs domains.
International Journal of Mathematics,
Vol. 35,
Issue. 10,
Tang, Yanyan
Tu, Zhenhan
and
Zhang, Shuo
2024.
Weak-type estimates of the Bergman projection on a class of singular Reinhardt domains.
Journal of Mathematical Analysis and Applications,
Vol. 539,
Issue. 2,
p.
128626.
Chakrabarti, Debraj
Tang, Yanyan
and
Zhang, Shuo
2025.
Toeplitz operators on monomial polyhedra.
Annali di Matematica Pura ed Applicata (1923 -),
Vol. 204,
Issue. 2,
p.
543.
Qin, Chuan
Wang, Maofa
and
Zhang, Shuo
2025.
$$L^p$$-norm Estimates of the Bergman Projection on the Monomial Polyhedra.
The Journal of Geometric Analysis,
Vol. 35,
Issue. 3,
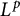 $L^p$-regularity of the Bergman projection on quotient domains
$L^p$-regularity of the Bergman projection on quotient domains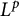 $L^p$
-boundedness for domains in a wide class of Reinhardt domains representable as sublevel sets of monomials, by expressing them as quotients of simpler domains. We prove a general transformation law relating
$L^p$
-boundedness for domains in a wide class of Reinhardt domains representable as sublevel sets of monomials, by expressing them as quotients of simpler domains. We prove a general transformation law relating 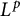 $L^p$
-boundedness on a domain and its quotient by a finite group. The range of p for which the Bergman projection is
$L^p$
-boundedness on a domain and its quotient by a finite group. The range of p for which the Bergman projection is 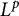 $L^p$
-bounded on our class of Reinhardt domains is found to shrink as the complexity of the domain increases .
$L^p$
-bounded on our class of Reinhardt domains is found to shrink as the complexity of the domain increases .