Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Tringali, Salvatore
2019.
Structural properties of subadditive families with applications to factorization theory.
Israel Journal of Mathematics,
Vol. 234,
Issue. 1,
p.
1.
ZHONG, QINGHAI
2020.
ON THE ARITHMETIC OF MORI MONOIDS AND DOMAINS.
Glasgow Mathematical Journal,
Vol. 62,
Issue. 2,
p.
313.
Geroldinger, Alfred
Gotti, Felix
and
Tringali, Salvatore
2021.
On strongly primary monoids, with a focus on Puiseux monoids.
Journal of Algebra,
Vol. 567,
Issue. ,
p.
310.
Bashir, Aqsa
Geroldinger, Alfred
and
Reinhart, Andreas
2021.
On the arithmetic of stable domains.
Communications in Algebra,
Vol. 49,
Issue. 11,
p.
4763.
Bashir, Aqsa
Geroldinger, Alfred
and
Zhong, Qinghai
2021.
Combinatorial and Additive Number Theory IV.
Vol. 347,
Issue. ,
p.
11.
Garcia, Stephan Ramon
Omar, Mohamed
O'Neill, Christopher
and
Yih, Samuel
2021.
Factorization length distribution for affine semigroups II: Asymptotic behavior for numerical semigroups with arbitrarily many generators.
Journal of Combinatorial Theory, Series A,
Vol. 178,
Issue. ,
p.
105358.
Edmonds, Ranthony A. C.
and
Juett, J. R.
2021.
Associates, irreducibility, and factorization length in monoid rings with zero divisors.
Communications in Algebra,
Vol. 49,
Issue. 5,
p.
1836.
Geroldinger, Alfred
Grynkiewicz, David J.
Oh, Jun Seok
and
Zhong, Qinghai
2022.
On product-one sequences over dihedral groups.
Journal of Algebra and Its Applications,
Vol. 21,
Issue. 04,
Grynkiewicz, David J.
2022.
The Characterization of Finite Elasticities.
Vol. 2316,
Issue. ,
p.
1.
Jiang, Nancy
Li, Bangzheng
and
Zhu, Sophie
2023.
On the primality and elasticity of algebraic valuations of cyclic free semirings.
International Journal of Algebra and Computation,
Vol. 33,
Issue. 02,
p.
197.
 $H$, let
$H$, let 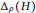 ${{\Delta }_{\rho }}\left( H \right)$ be the set of all positive integers d that occur as differences of arbitrarily long arithmetical progressions contained in sets of lengths havingmaximal elasticity
${{\Delta }_{\rho }}\left( H \right)$ be the set of all positive integers d that occur as differences of arbitrarily long arithmetical progressions contained in sets of lengths havingmaximal elasticity 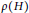 $\rho \left( H \right)$. We study
$\rho \left( H \right)$. We study 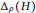 ${{\Delta }_{\rho }}\left( H \right)$ for transfer Krull monoids of finite type (including commutative Krull domains with finite class group) with methods from additive combinatorics, and also for a class of weakly Krull domains (including orders in algebraic number fields) for which we use ideal theoretic methods.
${{\Delta }_{\rho }}\left( H \right)$ for transfer Krull monoids of finite type (including commutative Krull domains with finite class group) with methods from additive combinatorics, and also for a class of weakly Krull domains (including orders in algebraic number fields) for which we use ideal theoretic methods.