Article contents
Haar Null Sets and the Consistent Reflection of Non-meagreness
Published online by Cambridge University Press: 20 November 2018
Abstract
A subset 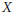 $X$ of a Polish group
$X$ of a Polish group  $G$ is called Haar null if there exist a Borel set
$G$ is called Haar null if there exist a Borel set  $B\,\supset \,X$ and Borel probability measure
$B\,\supset \,X$ and Borel probability measure 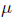 $\mu$ on
$\mu$ on  $G$ such that
$G$ such that 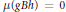 $\mu \left( g\,Bh \right)\,=\,0$ for every
$\mu \left( g\,Bh \right)\,=\,0$ for every 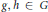 $g,\,h\,\in \,G$ . We prove that there exist a set
$g,\,h\,\in \,G$ . We prove that there exist a set 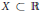 $X\,\subset \,\text{R}$ that is not Lebesgue null and a Borel probability measure
$X\,\subset \,\text{R}$ that is not Lebesgue null and a Borel probability measure 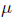 $\mu$ such that
$\mu$ such that 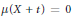 $\mu \left( X\,+\,t \right)\,=\,0$ for every
$\mu \left( X\,+\,t \right)\,=\,0$ for every 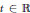 $t\,\in \,\text{R}$ . This answers a question from David Fremlin’s problem list by showing that one cannot simplify the definition of a Haar null set by leaving out the Borel set
$t\,\in \,\text{R}$ . This answers a question from David Fremlin’s problem list by showing that one cannot simplify the definition of a Haar null set by leaving out the Borel set  $B$ . (The answer was already known assuming the Continuum Hypothesis.)
$B$ . (The answer was already known assuming the Continuum Hypothesis.)
This result motivates the following Baire category analogue. It is consistent with 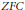 $ZFC$ that there exist an abelian Polish group
$ZFC$ that there exist an abelian Polish group  $G$ and a Cantor set
$G$ and a Cantor set 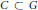 $C\,\subset \,G$ such that for every non-meagre set
$C\,\subset \,G$ such that for every non-meagre set 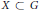 $X\,\subset \,\text{G}$ there exists a
$X\,\subset \,\text{G}$ there exists a 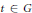 $t\in \text{G}$ such that
$t\in \text{G}$ such that  $C\,\cap \,\left( X\,+\,t \right)$ is relatively non-meagre in
$C\,\cap \,\left( X\,+\,t \right)$ is relatively non-meagre in  $C$ . This essentially generalizes results of Bartoszyński and Burke–Miller.
$C$ . This essentially generalizes results of Bartoszyński and Burke–Miller.
Keywords
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2014
References
- 4
- Cited by

