No CrossRef data available.
Article contents
Green Function and Self-adjoint Laplacians on Polyhedral Surfaces
Part of:
Deformations of analytic structures
Partial differential equations on manifolds; differential operators
Riemann surfaces
Spectral theory and eigenvalue problems
Published online by Cambridge University Press: 02 July 2019
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
Using Roelcke’s formula for the Green function, we explicitly construct a basis in the kernel of the adjoint Laplacian on a compact polyhedral surface 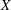 $X$ and compute the
$X$ and compute the 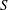 $S$-matrix of
$S$-matrix of 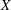 $X$ at the zero value of the spectral parameter. We apply these results to study various self-adjoint extensions of a symmetric Laplacian on a compact polyhedral surface of genus two with a single conical point. It turns out that the behaviour of the
$X$ at the zero value of the spectral parameter. We apply these results to study various self-adjoint extensions of a symmetric Laplacian on a compact polyhedral surface of genus two with a single conical point. It turns out that the behaviour of the  $S$-matrix at the zero value of the spectral parameter is sensitive to the geometry of the polyhedron.
$S$-matrix at the zero value of the spectral parameter is sensitive to the geometry of the polyhedron.
MSC classification
Information
- Type
- Article
- Information
- Copyright
- © Canadian Mathematical Society 2019
References
Aissiou, T., Hillairet, L., and Kokotov, A., Determinants of pseudo-laplacians. Math. Res. Lett. 19(2012), no. 6, 1297–1308. https://doi.org/10.4310/MRL.2012.v19.n6.a10CrossRefGoogle Scholar
Akhiezer, N. I. and Glazman, I. M., Theory of linear operators in Hilbert space. (vol. 1–2, Monographs and Studies in Mathematics, 9. Pitman (Advanced Publishing Program), Boston, Mass.-London, 1981.Google Scholar
Albeverio, S., Gesztesy, F., Hoegh-Krohn, R., and Holden, H., Solvable models in quantum mechanics. Second ed., AMS Chelsea Publishing, Providence, RI, 2005.Google Scholar
Berezin, F. and Shubin, M., The Schrödinger equation. In: Mathematics and its Applications (Soviet Series), 66. Kluwer Academic Publishers, 1991. https://doi.org/10.1007/978-94-011-3154-4Google Scholar
Colin De Verdiere, Y., Pseudo-laplaciens. I. Ann. Inst. Fourier 32(1982), 275–286.CrossRefGoogle Scholar
Farkas, H. and Kra, I., Riemann surfaces. In: Graduate Texts in Mathematics, 71. Springer-Verlag, New York-Berlin, 1980.Google Scholar
Fay, J., Theta functions on Riemann surfaces. In: Lecture notes in Mathematics, 352. Springer-Verlag, Berlin-New York, 1973.Google Scholar
Fay, J., Kernel functions, analytic torsion and moduli spaces. Mem. Amer. Math. Soc. 96(1992), no. 464. https://doi.org/10.1090/memo/0464Google Scholar
Hillairet, L., Spectral theory of translation surfaces: a short introduction. In: Actes du Séminaire de Théorie Spectrale et Géometrie, 28. Année 2009-2010, Sémin. Théor. Spectr. Géom., 28, Univ. Grenoble I, Saint-Martin-d’Hères, 2010, pp. 51–62.https://doi.org/10.5802/tsg.278Google Scholar
Hillairet, L. and Kokotov, A., Krein formula and S-matrix for Euclidean surfaces with conical singularities. J. Geom. Anal. 23(2013), no. 3, 1498–1529. https://doi.org/10.1007/s12220-012-9295-3CrossRefGoogle Scholar
Hillairet, L. and Kokotov, A., Isospectrality, comparison formulas for determinants of Laplacian and flat metrics with non-trivial holonomy. Proc. Amer. Math. Soc. 145(2017), 3915–3928. https://doi.org/10.1090/proc/13494CrossRefGoogle Scholar
Hillairet, L., Kalvin, V., and Kokotov, A., Moduli spaces of meromorphic functions and determinant of Laplacian. Trans. Amer. Math. Soc. 370(2018), 4559–4599. https://doi.org/10.1090/tran/7430CrossRefGoogle Scholar
Kay, B. S. and Studer, U. M., Boundary conditions for quantum mechanics on cones and fields around cosmic strings. Comm. Math. Phys. 139(1991), 103–139.CrossRefGoogle Scholar
Kirsten, K., Loya, P., and Park, J., Functional determinants for general self-adjoint extensions of Laplace type operators resulting from the generalized cone. Manuscripta Math. 125(2008), 95–126. https://doi.org/10.1007/s00229-007-0142-yCrossRefGoogle Scholar
Kokotov, A., Polyhedral surfaces and determinant of Laplacian. Proc. Amer. Math. Soc. 141(2013), 725–735. https://doi.org/10.1090/S0002-9939-2012-11531-XCrossRefGoogle Scholar
Kokotov, A. and Korotkin, D., Tau-functions on spaces of Abelian differentials and higher genus generalization of Ray-Singer formula. J. Differential Geom. 82(2009), no. 1, 35–100.CrossRefGoogle Scholar
Kondratjev, V. A., Boundary value problems for elliptic equations in domains with conical and angle points. Proc. Moscow Math. Soc. 16(1967), 209–292.Google Scholar
Lebedev, N. N., Special functions and their applications. In: Dover Books on Mathematics. reprint of 1965 ed.Google Scholar
Loya, P., McDonald, P., and Park, J., Zeta regularized determinants for conic manifolds. J. Funct. Anal. 242(2007), 195–229. https://doi.org/10.1016/j.jfa.2006.04.014CrossRefGoogle Scholar
Lukzen, E. N. and Shafarevich, A. I., On the kernel of the Laplace operator on two-dimensional polyhedra. Russ. J. Math. Phys. 24(2017), 508–514. https://doi.org/10.1134/S1061920817040070CrossRefGoogle Scholar
Maz’ya, V., Nazarov, S., and Plamenevskij, B., Asymptotic theory of elliptic boundary value problems in singularly perturbed domains. In: Operator Theory: Advances and Applications, 112. Birkhäuser Verlag, Basel, 2000.Google Scholar
Maz’ya, V. and Plamenevskij, B., Asymptotic behavior of the fundamental solutions of elliptic boundary value problems in domains with conical points. In: Probl. Mat. Anal., 7. Leningrad. Univ., Leningrad, 1979, pp. 100–145.Google Scholar
McMullen, C. T., Billiards and Teichmuller curves on Hiilbert modular surfaces. J. Amer. Math. Soc. 16(2003), 857–885. https://doi.org/10.1090/S0894-0347-03-00432-6CrossRefGoogle Scholar
Mooers, E. A., Heat kernel asymptotics on manifolds with conic singularities. J. Anal. Math. 78(1999), 1–36. https://doi.org/10.1007/BF02791127CrossRefGoogle Scholar
Nazarov, S. and Plamenevsky, B., Elliptic problems in domains with piecewise smooth boundaries. In: de Gruyter Expositions in Mathematics, 13. de Gruyter, Berlin, 1994. https://doi.org/10.1515/9783110848915.525Google Scholar
Okikiolu, K., Extremals for logarithmic Hardy-Littlewood-Sobolev inequalities on compact manifolds. Geom. Funct. Anal. 17(2008), 1655–1684. https://doi.org/10.1007/s00039-007-0636-5CrossRefGoogle Scholar
Polyakov, A. M., Quantum geometry of bosonic strings. Phys. Lett. B 103(1981), 207–210. https://doi.org/10.1016/0370-2693(81)90743-7CrossRefGoogle Scholar
Reed, M. and Simon, B., Methods of modern mathematical physics. II. In: Fourier analysis, self-adjointness. Academic Press/Harcourt Brace, New York-London, 1975.Google Scholar
Schiffer, M. and Spencer, D., Functionals of finite Riemann surfaces. Princeton University Press, Princeton, NJ, 1954.Google Scholar
Steiner, J., A geometrical mass and its extremal properties for metrics on S 2. Duke Math. J. 129(2005), 63–86. https://doi.org/10.1215/S0012-7094-04-12913-6CrossRefGoogle Scholar
Taylor, M. E., Partial differential equations. I. In: Basic Theory. Springer, New York, 1999.Google Scholar
Troyanov, M., Les surfaces euclidiennes à singularités coniques. Enseign. Math. 32(1968), 79–94.Google Scholar
Tyurin, A. N., Periods of quadratic differentials. Russian Math. Surveys 33(1978), 149–195, 272.CrossRefGoogle Scholar
Verlinde, E. and Verlinde, H., Chiral bosonization, determinants and the string partition function. Nuclear Phys. B 288(1987), 357–396. https://doi.org/10.1016/0550-3213(87)90219-7CrossRefGoogle Scholar


