Article contents
Gosset Polytopes in Picard Groups of del Pezzo Surfaces
Published online by Cambridge University Press: 20 November 2018
Abstract
In this article, we study the correspondence between the geometry of del Pezzo surfaces 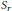 ${{s}_{r}}$ and the geometry of the
${{s}_{r}}$ and the geometry of the  $r$ -dimensional Gosset polytopes (
$r$ -dimensional Gosset polytopes ( 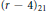 ${{(r-4)}_{21}}$ . We construct Gosset polytopes
${{(r-4)}_{21}}$ . We construct Gosset polytopes 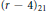 ${{(r-4)}_{21}}$ in Pic
${{(r-4)}_{21}}$ in Pic 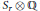 ${{S}_{r}}\,\otimes \,\mathbb{Q}$ whose vertices are lines, and we identify divisor classes in Pic
${{S}_{r}}\,\otimes \,\mathbb{Q}$ whose vertices are lines, and we identify divisor classes in Pic 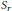 ${{s}_{r}}$ corresponding to
${{s}_{r}}$ corresponding to 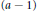 $(a-1)$ -simplexes
$(a-1)$ -simplexes  $(a\le r)$ ,
$(a\le r)$ , 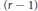 $(r-1)$ -simplexes and
$(r-1)$ -simplexes and 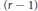 $(r-1)$ -crosspolytopes of the polytope
$(r-1)$ -crosspolytopes of the polytope 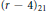 ${{(r-4)}_{21}}$ . Then we explain how these classes correspond to skew
${{(r-4)}_{21}}$ . Then we explain how these classes correspond to skew  $a$ -lines
$a$ -lines  $(a\le r)$ , exceptional systems, and rulings, respectively.
$(a\le r)$ , exceptional systems, and rulings, respectively.
As an application, we work on the monoidal transform for lines to study the local geometry of the polytope 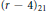 ${{(r-4)}_{21}}$ . And we show that the Gieser transformation and the Bertini transformation induce a symmetry of polytopes
${{(r-4)}_{21}}$ . And we show that the Gieser transformation and the Bertini transformation induce a symmetry of polytopes 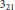 ${{3}_{21}}$ and
${{3}_{21}}$ and 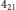 ${{4}_{21}}$ , respectively.
${{4}_{21}}$ , respectively.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2012
References
- 7
- Cited by

