Published online by Cambridge University Press: 20 November 2018
Permutation products and their various “fat diagonal” subspaces are studied from the topological and geometric points of view. We describe in detail the stabilizer and orbit stratifications related to the permutation action, producing a sharp upper bound for its depth and then paying particular attention to the geometry of the diagonal stratum. We exhibit an expression for the fundamental group of any permutation product of a connected space 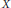 $X$ having the homotopy type of a CW complex in terms of
$X$ having the homotopy type of a CW complex in terms of 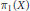 ${{\pi }_{1}}(X)$ and
${{\pi }_{1}}(X)$ and 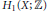 ${{H}_{1}}(X;\,\mathbb{Z})$. We then prove that the fundamental group of the configuration space of
${{H}_{1}}(X;\,\mathbb{Z})$. We then prove that the fundamental group of the configuration space of  $n$-points on
$n$-points on 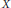 $X$, of which multiplicities do not exceed
$X$, of which multiplicities do not exceed  $n/2$, coincides with
$n/2$, coincides with 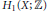 ${{H}_{1}}(X;\,\mathbb{Z})$. Further results consist in giving conditions for when fat diagonal subspaces of manifolds can be manifolds again. Various examples and homological calculations are included.
${{H}_{1}}(X;\,\mathbb{Z})$. Further results consist in giving conditions for when fat diagonal subspaces of manifolds can be manifolds again. Various examples and homological calculations are included.