Article contents
Extensions by Simple C*-Algebras: Quasidiagonal Extensions
Published online by Cambridge University Press: 20 November 2018
Abstract
Let  $A$ be an amenable separable
$A$ be an amenable separable  ${{C}^{*}}$ -algebra and
${{C}^{*}}$ -algebra and  $B$ be a non-unital but
$B$ be a non-unital but  $\sigma $ -unital simple
$\sigma $ -unital simple  ${{C}^{*}}$ - algebra with continuous scale. We show that two essential extensions
${{C}^{*}}$ - algebra with continuous scale. We show that two essential extensions  ${{\tau }_{1}}$ and
${{\tau }_{1}}$ and  ${{\tau }_{2}}$ of
${{\tau }_{2}}$ of  $A$ by
$A$ by  $B$ are approximately unitarily equivalent if and only if
$B$ are approximately unitarily equivalent if and only if
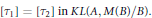 $$\left[ {{\tau }_{1}} \right]\,=\,\left[ {{\tau }_{2}} \right]\,\text{in}\,KL\left( A,\,M\left( B \right)/B \right).$$
$$\left[ {{\tau }_{1}} \right]\,=\,\left[ {{\tau }_{2}} \right]\,\text{in}\,KL\left( A,\,M\left( B \right)/B \right).$$
If  $A$ is assumed to satisfy the Universal Coefficient Theorem, there is a bijection from approximate unitary equivalence classes of the above mentioned extensions to
$A$ is assumed to satisfy the Universal Coefficient Theorem, there is a bijection from approximate unitary equivalence classes of the above mentioned extensions to 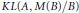 $KL\left( A,\,M\left( B \right)/B \right)$ . Using
$KL\left( A,\,M\left( B \right)/B \right)$ . Using 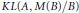 $KL\left( A,\,M\left( B \right)/B \right)$ , we compute exactly when an essential extension is quasidiagonal. We show that quasidiagonal extensions may not be approximately trivial. We also study the approximately trivial extensions.
$KL\left( A,\,M\left( B \right)/B \right)$ , we compute exactly when an essential extension is quasidiagonal. We show that quasidiagonal extensions may not be approximately trivial. We also study the approximately trivial extensions.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2005
References
- 8
- Cited by

