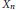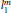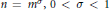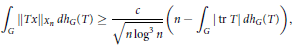Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Mankiewicz, Piotr
and
Tomczak-Jaegermann, Nicole
2003.
Vol. 2,
Issue. ,
p.
1201.
Wojtaszczyk, P.
2010.
Stability and Instance Optimality for Gaussian Measurements in Compressed Sensing.
Foundations of Computational Mathematics,
Vol. 10,
Issue. 1,
p.
1.