 $H$-categories and Co-
$H$-categories and Co-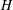 $H$-categories
$H$-categoriesPublished online by Cambridge University Press: 06 August 2019
Let 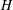 $H$ be a Hopf algebra. We consider
$H$ be a Hopf algebra. We consider 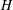 $H$-equivariant modules over a Hopf module category
$H$-equivariant modules over a Hopf module category 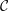 ${\mathcal{C}}$ as modules over the smash extension
${\mathcal{C}}$ as modules over the smash extension 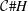 ${\mathcal{C}}\#H$. We construct Grothendieck spectral sequences for the cohomologies as well as the
${\mathcal{C}}\#H$. We construct Grothendieck spectral sequences for the cohomologies as well as the 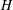 $H$-locally finite cohomologies of these objects. We also introduce relative
$H$-locally finite cohomologies of these objects. We also introduce relative  $({\mathcal{D}},H)$-Hopf modules over a Hopf comodule category
$({\mathcal{D}},H)$-Hopf modules over a Hopf comodule category  ${\mathcal{D}}$. These generalize relative
${\mathcal{D}}$. These generalize relative 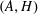 $(A,H)$-Hopf modules over an
$(A,H)$-Hopf modules over an 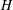 $H$-comodule algebra
$H$-comodule algebra  $A$. We construct Grothendieck spectral sequences for their cohomologies by using their rational
$A$. We construct Grothendieck spectral sequences for their cohomologies by using their rational 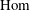 $\text{Hom}$ objects and higher derived functors of coinvariants.
$\text{Hom}$ objects and higher derived functors of coinvariants.
Author M.B. was supported by SERB Fellowship PDF/2017/000229. Author A.B. was partially supported by SERB Matrics fellowship MTR/2017/000112.