No CrossRef data available.
Published online by Cambridge University Press: 20 November 2018
The space now known as complete Erdős space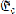 ${{\mathfrak{E}}_{\text{c}}}$ was introduced by Paul Erdős in 1940 as the closed subspace of the Hilbert space
${{\mathfrak{E}}_{\text{c}}}$ was introduced by Paul Erdős in 1940 as the closed subspace of the Hilbert space 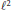 ${{\ell }^{2}}$ consisting of all vectors such that every coordinate is in the convergent sequence
${{\ell }^{2}}$ consisting of all vectors such that every coordinate is in the convergent sequence 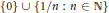 $\left\{ 0 \right\}\cup \left\{ 1/n:n\in \mathbb{N}\ \right\}$. In a solution to a problem posed by Lex
$\left\{ 0 \right\}\cup \left\{ 1/n:n\in \mathbb{N}\ \right\}$. In a solution to a problem posed by Lex 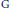 $G$. Oversteegen we present simple and useful topological characterizations of
$G$. Oversteegen we present simple and useful topological characterizations of 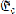 ${{\mathfrak{E}}_{\text{c}}}$. As an application we determine the class of factors of
${{\mathfrak{E}}_{\text{c}}}$. As an application we determine the class of factors of 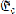 ${{\mathfrak{E}}_{\text{c}}}$. In another application we determine precisely which of the spaces that can be constructed in the Banach spaces
${{\mathfrak{E}}_{\text{c}}}$. In another application we determine precisely which of the spaces that can be constructed in the Banach spaces 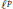 ${{\ell }^{p}}$ according to the ‘Erdős method’ are homeomorphic to
${{\ell }^{p}}$ according to the ‘Erdős method’ are homeomorphic to 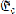 ${{\mathfrak{E}}_{\text{c}}}$. A novel application states that if
${{\mathfrak{E}}_{\text{c}}}$. A novel application states that if  $I$ is a Polishable
$I$ is a Polishable 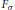 ${{F}_{\sigma }}$-ideal on
${{F}_{\sigma }}$-ideal on  $\omega $, then
$\omega $, then  $I$ with the Polish topology is homeomorphic to either
$I$ with the Polish topology is homeomorphic to either  $\mathbb{Z}$, the Cantor set
$\mathbb{Z}$, the Cantor set 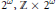 ${{2}^{\omega }},\,\mathbb{Z}\,\times \,{{2}^{\omega }}$, or
${{2}^{\omega }},\,\mathbb{Z}\,\times \,{{2}^{\omega }}$, or 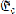 ${{\mathfrak{E}}_{\text{c}}}$. This last result answers a question that was asked by Stevo Todorčević.
${{\mathfrak{E}}_{\text{c}}}$. This last result answers a question that was asked by Stevo Todorčević.