Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Davison, J. L.
and
Shallit, J. O.
1991.
Continued fractions for some alternating series.
Monatshefte f�r Mathematik,
Vol. 111,
Issue. 2,
p.
119.
GRITZMANN, Peter
and
WILLS, Jörg M.
1993.
Handbook of Convex Geometry.
p.
765.
Poonen, Bjorn
and
Rodriguez-Villegas, Fernando
2000.
Lattice Polygons and the Number 12.
The American Mathematical Monthly,
Vol. 107,
Issue. 3,
p.
238.
Pikhurko, Oleg
2001.
Lattice points in lattice polytopes.
Mathematika,
Vol. 48,
Issue. 1-2,
p.
15.
Debarre, Olivier
2003.
Higher Dimensional Varieties and Rational Points.
Vol. 12,
Issue. ,
p.
93.
Böröczky, Károly
2005.
The stability of the Rogers–Shephard inequality and of some related inequalities.
Advances in Mathematics,
Vol. 190,
Issue. 1,
p.
47.
Darte, A.
Schreiber, R.
and
Villard, G.
2005.
Lattice-Based Memory Allocation.
IEEE Transactions on Computers,
Vol. 54,
Issue. 10,
p.
1242.
Henk, Martin
Schürmann, Achill
and
Wills, Jörg M.
2005.
Ehrhart Polynomials and Successive Minima.
Mathematika,
Vol. 52,
Issue. 1-2,
p.
1.
Nill, Benjamin
2005.
Gorenstein toric Fano varieties.
manuscripta mathematica,
Vol. 116,
Issue. 2,
p.
183.
Nill, Benjamin
2008.
Lattice polytopes having h∗-polynomials with given degree and linear coefficient.
European Journal of Combinatorics,
Vol. 29,
Issue. 7,
p.
1596.
Haase, Christian
Nill, Benjamin
and
Payne, Sam
2009.
Cayley decompositions of lattice polytopes and upper bounds for h*-polynomials.
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 2009,
Issue. 637,
Haase, Christian
and
Schicho, Josef
2009.
Lattice Polygons and the Number 2i + 7.
The American Mathematical Monthly,
Vol. 116,
Issue. 2,
p.
151.
Kasprzyk, Alexander M.
Kreuzer, Maximilian
and
Nill, Benjamin
2010.
On the combinatorial classification of toric log del Pezzo surfaces.
LMS Journal of Computation and Mathematics,
Vol. 13,
Issue. ,
p.
33.
Beck, Matthias
and
Stapledon, Alan
2010.
On the log-concavity of Hilbert series of Veronese subrings and Ehrhart series.
Mathematische Zeitschrift,
Vol. 264,
Issue. 1,
p.
195.
Treutlein, Jaron
2010.
Lattice polytopes of degree 2.
Journal of Combinatorial Theory, Series A,
Vol. 117,
Issue. 3,
p.
354.
LAGARIAS, JEFFREY C.
2010.
CYCLIC SYSTEMS OF SIMULTANEOUS CONGRUENCES.
International Journal of Number Theory,
Vol. 06,
Issue. 02,
p.
219.
Averkov, Gennadiy
Wagner, Christian
and
Weismantel, Robert
2011.
Maximal Lattice-Free Polyhedra: Finiteness and an Explicit Description in Dimension Three.
Mathematics of Operations Research,
Vol. 36,
Issue. 4,
p.
721.
Maydanskiy, Maksim
and
Mirabelli, Benjamin P.
2011.
Semisimplicity of the quantum cohomology for smooth Fano toric varieties associated with facet symmetric polytopes.
Electronic Research Announcements in Mathematical Sciences,
Vol. 18,
Issue. 0,
p.
131.
Liu, Heling
and
Zong, Chuanming
2011.
On the classification of convex lattice polytopes.
advg,
Vol. 11,
Issue. 4,
p.
711.
Nill, Benjamin
and
Ziegler, Günter M.
2011.
Projecting Lattice Polytopes Without Interior Lattice Points.
Mathematics of Operations Research,
Vol. 36,
Issue. 3,
p.
462.
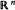 whose vertices are all in
whose vertices are all in 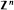 . The volume of a lattice polytope P containing exactly k ≥ 1 points in d
. The volume of a lattice polytope P containing exactly k ≥ 1 points in d 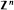 in its interior is bounded above by
in its interior is bounded above by 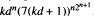 . Any lattice polytope in
. Any lattice polytope in 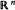 of volume V can after an integral unimodular transformation be contained in a lattice cube having side length at most n˙ n ! V. Thus the number of equivalence classes under integer unimodular transformations of lattice poly topes of bounded volume is finite. If S is any simplex of maximum volume inside a closed bounded convex body K in
of volume V can after an integral unimodular transformation be contained in a lattice cube having side length at most n˙ n ! V. Thus the number of equivalence classes under integer unimodular transformations of lattice poly topes of bounded volume is finite. If S is any simplex of maximum volume inside a closed bounded convex body K in 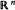 having nonempty interior, then K⊆ ( n + 2)S — (n+ l)s where mS denotes a nomothetic copy of S with scale factor m, and s is the centroid of S.
having nonempty interior, then K⊆ ( n + 2)S — (n+ l)s where mS denotes a nomothetic copy of S with scale factor m, and s is the centroid of S.
