Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Ramezanpour, M.
and
Barootkoob, S.
2017.
Generalized module extension Banach algebras: Derivations and weak amenability.
Quaestiones Mathematicae,
Vol. 40,
Issue. 4,
p.
451.
Javanshiri, Hossein
and
Nemati, Mehdi
2018.
Amalgamated duplication of the Banach algebra 𝔄 along a 𝔄-bimodule 𝒜.
Journal of Algebra and Its Applications,
Vol. 17,
Issue. 09,
p.
1850169.
Dabhi, Prakash A.
and
Upadhyay, Rakshit S.
2019.
The Semigroup Algebra
$$\ell ^1(\mathbb Z^2,\max )$$
ℓ
1
(
Z
2
,
max
)
is a Bochner–Schoenberg–Eberlein (BSE) Algebra.
Mediterranean Journal of Mathematics,
Vol. 16,
Issue. 1,
Abtahi, Fatemeh
Kamali, Zeinab
and
Toutounchi, Maryam
2019.
The Bochner-Schoenberg-Eberlein property for vector-valued Lipschitz algebras.
Journal of Mathematical Analysis and Applications,
Vol. 479,
Issue. 1,
p.
1172.
Ghasemi, M.
and
Mehdipour, M. J.
2021.
Derivations on Banach algebras of connected multiplicative linear functionals.
Bulletin of the Malaysian Mathematical Sciences Society,
Vol. 44,
Issue. 3,
p.
1727.
Abtahi, Fatemeh
2021.
-algebra structure on certain Banach algebra products.
Canadian Mathematical Bulletin,
Vol. 64,
Issue. 3,
p.
678.
ALIZADEH, NASRIN
EBADIAN, ALI
OSTADBASHI, SAEID
and
JABBARI, ALI
2022.
THE BOCHNER–SCHOENBERG-EBERLEIN PROPERTY OF EXTENSIONS OF BANACH ALGEBRAS AND BANACH MODULES.
Bulletin of the Australian Mathematical Society,
Vol. 105,
Issue. 1,
p.
134.
Ebadian, Ali
and
Jabbari, Ali
2022.
The Bochner–Schoenberg–Eberlein property for amalgamated duplication of Banach algebras.
Journal of Algebra and Its Applications,
Vol. 21,
Issue. 08,
Dabhi, Prakash A.
Patel, Savan K.
and
Pipaliya, Yuvraj D.
2023.
On various spectra of elements of $${\mathcal {A}} \times _\theta {\mathcal {B}}$$.
The Journal of Analysis,
Vol. 31,
Issue. 1,
p.
855.
Abtahi, Fatemeh
and
Pedaran, Ahmad
2023.
On characterizations of the image of Gelfand transform and BED property for vector valued ℓ−algebras.
Journal of Mathematical Analysis and Applications,
Vol. 517,
Issue. 1,
p.
126570.
Abolfathi, Mohammad Ali
Ebadian, Ali
and
Jabbari, Ali
2024.
The Bochner-Schoenberg-Eberlein module property for amalgamated duplication of Banach algebras.
Hacettepe Journal of Mathematics and Statistics,
Vol. 53,
Issue. 4,
p.
915.
Dabhi, Jekwin J.
and
Dabhi, Prakash A.
2024.
On the UC$$^*$$NP of the Lau product $${\mathcal {A}}\times _\theta {\mathcal {B}}$$.
Rendiconti del Circolo Matematico di Palermo Series 2,
Vol. 73,
Issue. 8,
p.
2949.
Abtahi, Fatemeh
Rejali, Ali
and
Sayaf, Farshad
2024.
The BSE property for some vector-valued Banach function algebras.
Acta Mathematica Scientia,
Vol. 44,
Issue. 5,
p.
1945.
Soleimani Malekan, Meisam
2025.
On characterizations of the image of the Gelfand transform of some Banach algebras of vector-valued functions.
The Journal of Analysis,
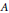 $A$ and
$A$ and 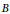 $B$ and a character
$B$ and a character  $\theta $ of
$\theta $ of 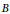 $B$ is a
certain Banach algebra product
$B$ is a
certain Banach algebra product 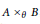 $A\,{{\times }_{\theta }}\,B$, which is a splitting extension of
$A\,{{\times }_{\theta }}\,B$, which is a splitting extension of 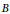 $B$ by
$B$ by 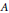 $A$. We investigate two topics for the algebra
$A$. We investigate two topics for the algebra 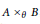 $A\,{{\times }_{\theta }}\,B$ in relation to the corresponding ones of
$A\,{{\times }_{\theta }}\,B$ in relation to the corresponding ones of 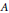 $A$ and
$A$ and 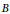 $B$. The first one is the Bochner–Schoenberg–Eberlein property and the algebra of Bochner–Schoenberg–Eberlein functions on the spectrum, whereas the second one concerns the wide range of spectral synthesis problems for
$B$. The first one is the Bochner–Schoenberg–Eberlein property and the algebra of Bochner–Schoenberg–Eberlein functions on the spectrum, whereas the second one concerns the wide range of spectral synthesis problems for 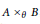 $A\,{{\times }_{\theta }}\,B$.
$A\,{{\times }_{\theta }}\,B$.