Published online by Cambridge University Press: 20 November 2018
For the  $q$-series
$q$-series 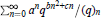 $\sum\nolimits_{n=0}^{\infty }{{{a}^{n}}{{q}^{b{{n}^{2}}+cn}}/}\,{{(q)}_{n}}$ we construct a companion
$\sum\nolimits_{n=0}^{\infty }{{{a}^{n}}{{q}^{b{{n}^{2}}+cn}}/}\,{{(q)}_{n}}$ we construct a companion  $q$-series such that the asymptotic expansions of their logarithms as
$q$-series such that the asymptotic expansions of their logarithms as 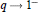 $q\,\to \,{{1}^{-}}$ differ only in the dominant few terms. The asymptotic expansion of their quotient then has a simple closed form; this gives rise to a new
$q\,\to \,{{1}^{-}}$ differ only in the dominant few terms. The asymptotic expansion of their quotient then has a simple closed form; this gives rise to a new  $q$–hypergeometric identity. We give an asymptotic expansion of a general class of
$q$–hypergeometric identity. We give an asymptotic expansion of a general class of  $q$-series containing some of Ramanujan's mock theta functions and Selberg's identities.
$q$-series containing some of Ramanujan's mock theta functions and Selberg's identities.