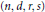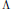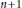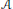Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Buczyńska, Weronika
Buczyński, Jarosław
Kleppe, Johannes
and
Teitler, Zach
2015.
Apolarity and direct sum decomposability of polynomials.
Michigan Mathematical Journal,
Vol. 64,
Issue. 4,