No CrossRef data available.
Article contents
Annihilators of the Ideal Class Group of a Cyclic Extension of an Imaginary Quadratic Field
Published online by Cambridge University Press: 09 January 2019
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
The aim of this paper is to study the group of elliptic units of a cyclic extension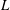 
$L$ of an imaginary quadratic field 
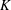$K$ such that the degree 
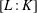$[L:K]$ is a power of an odd prime 
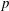$p$. We construct an explicit root of the usual top generator of this group, and we use it to obtain an annihilation result of the 
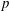$p$-Sylow subgroup of the ideal class group of 
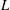$L$.
Keywords
MSC classification
Information
- Type
- Article
- Information
- Copyright
- © Canadian Mathematical Society 2018
Footnotes
The second author was supported under Project 15-15785S of the Czech Science Foundation.
References
Bley, W.,
Wild Euler systems of elliptic units and the equivariant Tamagawa number conjecture
. J. Reine Angew. Math.
577(2004), 117–146. https://doi.org/10.1515/crll.2004.2004.577.117.Google Scholar
Bley, W.,
Equivariant Tamagawa number conjecture for abelian extensions of a quadratic imaginary field
. Doc. Math.
11(2006), 73–118.Google Scholar
Burns, D.,
Congruences between derivatives of abelian L-functions at s = 0
. Invent. Math.
169(2007), 451–499. https://doi.org/10.1007/s00222-007-0052-3.Google Scholar
Gras, G.,
Class field theory. From theory to practice
. Springer Monographs in Mathematics, Springer-Verlag, Berlin, 2003.Google Scholar
Greither, C. and Kučera, R.,
Annihilators for the class group of a cyclic field of prime power degree
. Acta Arith.
112(2004), 177–198. https://doi.org/10.4064/aa112-2-6.Google Scholar
Greither, C. and Kučera, R.,
Annihilators for the class group of a cyclic field of prime power degree II
. Canad. J. Math.
58(2006), 580–599. https://doi.org/10.4153/CJM-2006-024-2.Google Scholar
Greither, C. and Kučera, R.,
Linear forms on Sinnott’s module
. J. Number Theory
141(2014), 324–342. https://doi.org/10.1016/j.jnt.2014.02.003.Google Scholar
Greither, C. and Kučera, R.,
Eigenspaces of the ideal class group
. Ann. Inst. Fourier (Grenoble)
64(2014), 2165–2203. https://doi.org/10.5802/aif.2908.Google Scholar
Greither, C. and Kučera, R.,
Annihilators for the class group of a cyclic field of prime power degree III
. Publ. Math. Debrecen
86(2015), no. 3–4, 401–421.Google Scholar
Ohshita, T.,
On higher Fitting ideals of Iwasawa modules of ideal class groups over imaginary quadratic fields and Euler systems of elliptic units
. Kyoto J. Math.
53(2013), 845–887. https://doi.org/10.1215/21562261-2366118.Google Scholar
Oukhaba, H.,
Index formulas for ramified elliptic units
. Compositio Math.
137(2003), 1–22. https://doi.org/10.1023/A:1023667807218.Google Scholar
Rubin, K.,
Global units and ideal class groups
. Invent. Math.
89(1987), 511–526. https://doi.org/10.1007/BF01388983.Google Scholar
Rubin, K.,
Stark units and Kolyvagin’s “Euler systems”
. J. Reine Angew. Math.
425(1992), 141–154. https://doi.org/10.1515/crll.1992.425.141.Google Scholar
Sinnott, W.,
On the Stickelberger ideal and the circular units of an abelian field
. Invent. Math.
62(1980), 181–234. https://doi.org/10.1007/BF01389158.Google Scholar
Thaine, F.,
On the ideal class groups of real abelian number fields
. Ann. of Math. (2)
128(1988), no. 1, 1–18. https://doi.org/10.2307/1971460.Google Scholar
You have
Access