No CrossRef data available.
Published online by Cambridge University Press: 20 August 2020
We prove a “splicing formula” for the LMO invariant, which is the universal finite-type invariant of rational homology three-spheres. Specifically, if a rational homology three-sphere M is obtained by gluing the exteriors of two framed knots 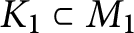 $K_1 \subset M_1$
and
$K_1 \subset M_1$
and
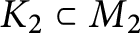 $K_2\subset M_2$
in rational homology three-spheres, our formula expresses the LMO invariant of M in terms of the Kontsevich–LMO invariants of
$K_2\subset M_2$
in rational homology three-spheres, our formula expresses the LMO invariant of M in terms of the Kontsevich–LMO invariants of
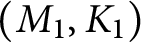 $(M_1,K_1)$
and
$(M_1,K_1)$
and
 $(M_2,K_2)$
. The proof uses the techniques that Bar-Natan and Lawrence developed to obtain a rational surgery formula for the LMO invariant. In low degrees, we recover Fujita’s formula for the Casson–Walker invariant, and we observe that the second term of the Ohtsuki series is not additive under “standard” splicing. The splicing formula also works when each
$(M_2,K_2)$
. The proof uses the techniques that Bar-Natan and Lawrence developed to obtain a rational surgery formula for the LMO invariant. In low degrees, we recover Fujita’s formula for the Casson–Walker invariant, and we observe that the second term of the Ohtsuki series is not additive under “standard” splicing. The splicing formula also works when each
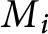 $M_i$
comes with a link
$M_i$
comes with a link
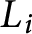 $L_i$
in addition to the knot
$L_i$
in addition to the knot
 $K_i$
, hence we get a “satellite formula” for the Kontsevich–LMO invariant.
$K_i$
, hence we get a “satellite formula” for the Kontsevich–LMO invariant.
This research has been funded by the project “ITIQ-3D” of the Région Bourgogne Franche–Comté. G.M. is partly supported by the project “AlMaRe” (ANR-19-CE40-0001-01) and by the EIPHI Graduate School (ANR-17-EURE-0002).