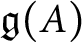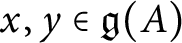Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Feingold, Alex J.
Kleinschmidt, Axel
and
Nicolai, Hermann
2024.
Decompositions of Hyperbolic Kac–Moody Algebras with Respect to Imaginary Root Groups.
Communications in Mathematical Physics,
Vol. 405,
Issue. 10,