No CrossRef data available.
Published online by Cambridge University Press: 17 March 2022
Let R be a discrete valuation domain with field of fractions Q and maximal ideal generated by
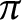 $\pi $
. Let
$\pi $
. Let
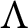 $\Lambda $
be an R-order such that
$\Lambda $
be an R-order such that
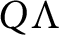 $Q\Lambda $
is a separable Q-algebra. Maranda showed that there exists
$Q\Lambda $
is a separable Q-algebra. Maranda showed that there exists
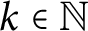 $k\in \mathbb {N}$
such that for all
$k\in \mathbb {N}$
such that for all
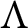 $\Lambda $
-lattices L and M, if
$\Lambda $
-lattices L and M, if
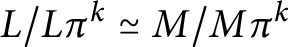 $L/L\pi ^k\simeq M/M\pi ^k$
, then
$L/L\pi ^k\simeq M/M\pi ^k$
, then
 $L\simeq M$
. Moreover, if R is complete and L is an indecomposable
$L\simeq M$
. Moreover, if R is complete and L is an indecomposable
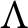 $\Lambda $
-lattice, then
$\Lambda $
-lattice, then
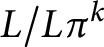 $L/L\pi ^k$
is also indecomposable. We extend Maranda’s theorem to the class of R-reduced R-torsion-free pure-injective
$L/L\pi ^k$
is also indecomposable. We extend Maranda’s theorem to the class of R-reduced R-torsion-free pure-injective
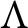 $\Lambda $
-modules.
$\Lambda $
-modules.
As an application of this extension, we show that if
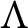 $\Lambda $
is an order over a Dedekind domain R with field of fractions Q such that
$\Lambda $
is an order over a Dedekind domain R with field of fractions Q such that
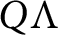 $Q\Lambda $
is separable, then the lattice of open subsets of the R-torsion-free part of the right Ziegler spectrum of
$Q\Lambda $
is separable, then the lattice of open subsets of the R-torsion-free part of the right Ziegler spectrum of
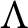 $\Lambda $
is isomorphic to the lattice of open subsets of the R-torsion-free part of the left Ziegler spectrum of
$\Lambda $
is isomorphic to the lattice of open subsets of the R-torsion-free part of the left Ziegler spectrum of
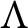 $\Lambda $
.
$\Lambda $
.
Furthermore, with k as in Maranda’s theorem, we show that if M is R-torsion-free and
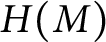 $H(M)$
is the pure-injective hull of M, then
$H(M)$
is the pure-injective hull of M, then
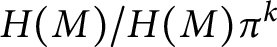 $H(M)/H(M)\pi ^k$
is the pure-injective hull of
$H(M)/H(M)\pi ^k$
is the pure-injective hull of
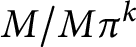 $M/M\pi ^k$
. We use this result to give a characterization of R-torsion-free pure-injective
$M/M\pi ^k$
. We use this result to give a characterization of R-torsion-free pure-injective
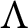 $\Lambda $
-modules and describe the pure-injective hulls of certain R-torsion-free
$\Lambda $
-modules and describe the pure-injective hulls of certain R-torsion-free
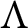 $\Lambda $
-modules.
$\Lambda $
-modules.
The majority of this work was completed while the author was employed by the University of Camerino.