No CrossRef data available.
Published online by Cambridge University Press: 03 November 2021
We investigate the notion of relatively amenable topological action and show that the action of Thompson’s group T on
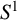 $S^1$
is relatively amenable with respect to Thompson’s group F. We use this to conclude that F is exact if and only if T is exact. Moreover, we prove that the groupoid of germs of the action of T on
$S^1$
is relatively amenable with respect to Thompson’s group F. We use this to conclude that F is exact if and only if T is exact. Moreover, we prove that the groupoid of germs of the action of T on
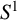 $S^1$
is Borel amenable.
$S^1$
is Borel amenable.