Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Ciepliński, Krzysztof
2020.
Ulam Stability of a Functional Equation in Various Normed Spaces.
Symmetry,
Vol. 12,
Issue. 7,
p.
1119.
Bahyrycz, Anna
Brzdęk, Janusz
El-hady, El-sayed
and
Leśniak, Zbigniew
2021.
On Ulam Stability of Functional Equations in 2-Normed Spaces—A Survey.
Symmetry,
Vol. 13,
Issue. 11,
p.
2200.
Ciepliński, Krzysztof
2021.
Ulam stability of functional equations in 2-Banach spaces via the fixed point method.
Journal of Fixed Point Theory and Applications,
Vol. 23,
Issue. 3,
Nuino, Ahmed
2021.
On the Brzdȩk’s fixed point approach to stability of a Drygas functional equation in 2-Banach spaces.
Journal of Fixed Point Theory and Applications,
Vol. 23,
Issue. 2,
El-hady, El-sayed
and
Brzdęk, Janusz
2022.
On Ulam Stability of Functional Equations in 2-Normed Spaces—A Survey II.
Symmetry,
Vol. 14,
Issue. 7,
p.
1365.
Sharma, Ravinder Kumar
and
Chandok, Sumit
2022.
The Generalized Hyperstability of General Linear Equation in Quasi-2-Banach Space.
Acta Mathematica Scientia,
Vol. 42,
Issue. 4,
p.
1357.
Sharma, Ravinder Kumar
Chandok, Sumit
and
Dey, Bikash Koli
2023.
Equivalence for Various Forms of Quadratic Functional Equations and Their Generalized Hyers–Ulam–Rassias Stability.
International Journal of Mathematics and Mathematical Sciences,
Vol. 2023,
Issue. 1,
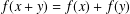 $f(x+y)=f(x)+f(y)$, but we believe that this method can be applied for many other equations. In particular we provide an extension of an earlier stability result that has been motivated by a problem of Th. M. Rassias. The main tool is a recent fixed point theorem in some spaces of functions with values in 2-Banach spaces.
$f(x+y)=f(x)+f(y)$, but we believe that this method can be applied for many other equations. In particular we provide an extension of an earlier stability result that has been motivated by a problem of Th. M. Rassias. The main tool is a recent fixed point theorem in some spaces of functions with values in 2-Banach spaces.