Published online by Cambridge University Press: 27 October 2015
Let 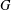 $G$ be a commutative group,
$G$ be a commutative group, 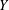 $Y$ a real Banach space and
$Y$ a real Banach space and  $f:G\rightarrow Y$. We prove the Ulam–Hyers stability theorem for the cyclic functional equation
$f:G\rightarrow Y$. We prove the Ulam–Hyers stability theorem for the cyclic functional equation  $$\begin{eqnarray}\displaystyle \frac{1}{|H|}\mathop{\sum }_{h\in H}f(x+h\cdot y)=f(x)+f(y) & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \frac{1}{|H|}\mathop{\sum }_{h\in H}f(x+h\cdot y)=f(x)+f(y) & & \displaystyle \nonumber\end{eqnarray}$$ $x,y\in {\rm\Omega}$, where
$x,y\in {\rm\Omega}$, where  $H$ is a finite cyclic subgroup of
$H$ is a finite cyclic subgroup of  $\text{Aut}(G)$ and
$\text{Aut}(G)$ and 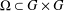 ${\rm\Omega}\subset G\times G$ satisfies a certain condition. As a consequence, we consider a measure zero stability problem of the functional equation
${\rm\Omega}\subset G\times G$ satisfies a certain condition. As a consequence, we consider a measure zero stability problem of the functional equation 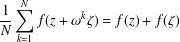 $$\begin{eqnarray}\displaystyle \frac{1}{N}\mathop{\sum }_{k=1}^{N}f(z+{\it\omega}^{k}{\it\zeta})=f(z)+f({\it\zeta}) & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \frac{1}{N}\mathop{\sum }_{k=1}^{N}f(z+{\it\omega}^{k}{\it\zeta})=f(z)+f({\it\zeta}) & & \displaystyle \nonumber\end{eqnarray}$$ $(z,{\it\zeta})\in {\rm\Omega}$, where
$(z,{\it\zeta})\in {\rm\Omega}$, where 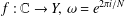 $f:\mathbb{C}\rightarrow Y,\,{\it\omega}=e^{2{\it\pi}i/N}$ and
$f:\mathbb{C}\rightarrow Y,\,{\it\omega}=e^{2{\it\pi}i/N}$ and  ${\rm\Omega}\subset \mathbb{C}^{2}$ has four-dimensional Lebesgue measure
${\rm\Omega}\subset \mathbb{C}^{2}$ has four-dimensional Lebesgue measure 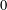 $0$.
$0$.