Article contents
A NOTE ON JEŚMANOWICZ’ CONJECTURE CONCERNING PRIMITIVE PYTHAGOREAN TRIPLES
Published online by Cambridge University Press: 26 September 2016
Abstract
Let  $a,b,c$ be a primitive Pythagorean triple and set
$a,b,c$ be a primitive Pythagorean triple and set 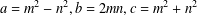 $a=m^{2}-n^{2},b=2mn,c=m^{2}+n^{2}$ , where
$a=m^{2}-n^{2},b=2mn,c=m^{2}+n^{2}$ , where  $m$ and
$m$ and  $n$ are positive integers with
$n$ are positive integers with  $m>n$ ,
$m>n$ , 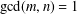 $\text{gcd}(m,n)=1$ and
$\text{gcd}(m,n)=1$ and 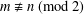 $m\not \equiv n~(\text{mod}~2)$ . In 1956, Jeśmanowicz conjectured that the only positive integer solution to the Diophantine equation
$m\not \equiv n~(\text{mod}~2)$ . In 1956, Jeśmanowicz conjectured that the only positive integer solution to the Diophantine equation 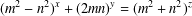 $(m^{2}-n^{2})^{x}+(2mn)^{y}=(m^{2}+n^{2})^{z}$ is
$(m^{2}-n^{2})^{x}+(2mn)^{y}=(m^{2}+n^{2})^{z}$ is 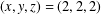 $(x,y,z)=(2,2,2)$ . We use biquadratic character theory to investigate the case with
$(x,y,z)=(2,2,2)$ . We use biquadratic character theory to investigate the case with 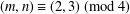 $(m,n)\equiv (2,3)~(\text{mod}~4)$ . We show that Jeśmanowicz’ conjecture is true in this case if
$(m,n)\equiv (2,3)~(\text{mod}~4)$ . We show that Jeśmanowicz’ conjecture is true in this case if 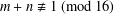 $m+n\not \equiv 1~(\text{mod}~16)$ or
$m+n\not \equiv 1~(\text{mod}~16)$ or 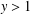 $y>1$ . Finally, using these results together with Laurent’s refinement of Baker’s theorem, we show that Jeśmanowicz’ conjecture is true if
$y>1$ . Finally, using these results together with Laurent’s refinement of Baker’s theorem, we show that Jeśmanowicz’ conjecture is true if 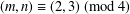 $(m,n)\equiv (2,3)~(\text{mod}~4)$ and
$(m,n)\equiv (2,3)~(\text{mod}~4)$ and 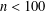 $n<100$ .
$n<100$ .
MSC classification
Information
- Type
- Research Article
- Information
- Copyright
- © 2016 Australian Mathematical Publishing Association Inc.
References
- 6
- Cited by

