Published online by Cambridge University Press: 03 May 2018
Let 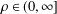 $\unicode[STIX]{x1D70C}\in (0,\infty ]$ be a real number. In this short note, we extend a recent result of Marques and Ramirez [‘On exceptional sets: the solution of a problem posed by K. Mahler’, Bull. Aust. Math. Soc.94 (2016), 15–19] by proving that any subset of
$\unicode[STIX]{x1D70C}\in (0,\infty ]$ be a real number. In this short note, we extend a recent result of Marques and Ramirez [‘On exceptional sets: the solution of a problem posed by K. Mahler’, Bull. Aust. Math. Soc.94 (2016), 15–19] by proving that any subset of 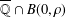 $\overline{\mathbb{Q}}\cap B(0,\unicode[STIX]{x1D70C})$, which is closed under complex conjugation and contains
$\overline{\mathbb{Q}}\cap B(0,\unicode[STIX]{x1D70C})$, which is closed under complex conjugation and contains 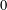 $0$, is the exceptional set of uncountably many analytic transcendental functions with rational coefficients and radius of convergence
$0$, is the exceptional set of uncountably many analytic transcendental functions with rational coefficients and radius of convergence 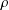 $\unicode[STIX]{x1D70C}$. This solves the question posed by K. Mahler completely.
$\unicode[STIX]{x1D70C}$. This solves the question posed by K. Mahler completely.
The authors were supported by CNPq-Brazil.