No CrossRef data available.
Article contents
HYPERGROUP ALGEBRAS AS TOPOLOGICAL ALGEBRAS
Published online by Cambridge University Press: 13 June 2014
Abstract
Let 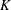 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}K$ be a locally compact hypergroup endowed with a left Haar measure and let
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}K$ be a locally compact hypergroup endowed with a left Haar measure and let 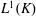 $L^1(K)$ be the usual Lebesgue space of
$L^1(K)$ be the usual Lebesgue space of 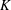 $K$ with respect to the left Haar measure. We investigate some properties of
$K$ with respect to the left Haar measure. We investigate some properties of  $L^1(K)$ under a locally convex topology
$L^1(K)$ under a locally convex topology 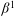 $\beta ^1$. Among other things, the semireflexivity of
$\beta ^1$. Among other things, the semireflexivity of  $(L^1(K), \beta ^1)$ and of sequentially
$(L^1(K), \beta ^1)$ and of sequentially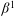 $\beta ^1$-continuous functionals is studied. We also show that
$\beta ^1$-continuous functionals is studied. We also show that 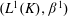 $(L^1(K), \beta ^1)$ with the convolution multiplication is always a complete semitopological algebra, whereas it is a topological algebra if and only if
$(L^1(K), \beta ^1)$ with the convolution multiplication is always a complete semitopological algebra, whereas it is a topological algebra if and only if 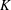 $K$ is compact.
$K$ is compact.
Information
- Type
- Research Article
- Information
- Bulletin of the Australian Mathematical Society , Volume 90 , Issue 3 , December 2014 , pp. 486 - 493
- Copyright
- Copyright © 2014 Australian Mathematical Publishing Association Inc.

