Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Hodel, R.E.
and
Vaughan, J.E.
1974.
A note on [a, b]-compactness.
General Topology and its Applications,
Vol. 4,
Issue. 2,
p.
179.
Smith, J. C.
1975.
Properties of weak 𝜃̄-refinable spaces.
Proceedings of the American Mathematical Society,
Vol. 53,
Issue. 2,
p.
511.
Smith, J. C.
1976.
A remark on irreducible spaces.
Proceedings of the American Mathematical Society,
Vol. 57,
Issue. 1,
p.
133.
Wicke, H. H.
and
Worrell, J. M.
1976.
Point-countability and compactness.
Proceedings of the American Mathematical Society,
Vol. 55,
Issue. 2,
p.
427.
Boone, James R.
1977.
Set-Theoretic Topology.
p.
73.
Blair, Robert L.
1977.
Set-Theoretic Topology.
p.
17.
Worrell, John M.
1977.
Set-Theoretic Topology.
p.
413.
Smith, J. C.
1979.
Applications of shrinkable covers.
Proceedings of the American Mathematical Society,
Vol. 73,
Issue. 3,
p.
379.
Junnila, Heikki J.K.
1980.
Surveys in General Topology.
p.
195.
Reilly, I. L.
and
Vamanamurthy, M. K.
1980.
On hereditarily Lindelöf spaces.
Bulletin of the Australian Mathematical Society,
Vol. 21,
Issue. 3,
p.
357.
Wicke, H.H.
and
Worrell, J.M.
1980.
Surveys in General Topology.
p.
495.
Förster, Ortwin
and
Grabner, Gary
1982.
The metacompactness of spaces with bases of subinfinite rank.
Topology and its Applications,
Vol. 13,
Issue. 2,
p.
115.
BURKE, Dennis K.
1984.
Handbook of Set-Theoretic Topology.
p.
347.
Balogh, Zoltán
1986.
Paracompactness in normal, locally connected, rim-compact spaces.
Topology and its Applications,
Vol. 22,
Issue. 1,
p.
1.
Tong, Jingcheng
1988.
On decomposition of proper compactness in fuzzy topological spaces.
Journal of Mathematical Analysis and Applications,
Vol. 134,
Issue. 1,
p.
46.
Gale, Sherry L.
1992.
Measure-compact spaces.
Topology and its Applications,
Vol. 45,
Issue. 2,
p.
103.
Yajima, Yukinobu
1998.
Analogous results to two classical characterizations of covering properties by products.
Topology and its Applications,
Vol. 84,
Issue. 1-3,
p.
3.
Ergun, N.
and
Noiri, T.
2001.
On Finally Compact Spaces.
Acta Mathematica Hungarica,
Vol. 92,
Issue. 1-2,
p.
61.
Arhangel'skii, Alexander V.
2005.
D-spaces and covering properties.
Topology and its Applications,
Vol. 146-147,
Issue. ,
p.
437.
Bennett, Harold
Byerly, Robert
and
Lutzer, David
2006.
Compact Gδ sets.
Topology and its Applications,
Vol. 153,
Issue. 12,
p.
2169.
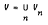 where each Vn covers X and for x є X there exists n such that Vn is of countable order at x. We replace point countable open cover by a δθ-cover in Aquaro's Theorem and also generalize the result of Worrell and Wicke that a θ-refinable countably compact space is compact and Jones′ result that ℵ1-compact Moore space is Lindelöf which was used to prove his classic result that a normal separable Moore space is metrizable, using the continuum hypothesis.
where each Vn covers X and for x є X there exists n such that Vn is of countable order at x. We replace point countable open cover by a δθ-cover in Aquaro's Theorem and also generalize the result of Worrell and Wicke that a θ-refinable countably compact space is compact and Jones′ result that ℵ1-compact Moore space is Lindelöf which was used to prove his classic result that a normal separable Moore space is metrizable, using the continuum hypothesis.
