Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Morris, Sidney A.
1973.
Varieties of topological groups and left adjoint functors.
Journal of the Australian Mathematical Society,
Vol. 16,
Issue. 2,
p.
220.
Morris, Sidney A.
and
Thompson, H. B.
1974.
Free topological groups with no small subgroups.
Proceedings of the American Mathematical Society,
Vol. 46,
Issue. 3,
p.
431.
Ordman, Edward T.
1974.
Free products of topological groups which are 𝑘_{𝜔}-spaces.
Transactions of the American Mathematical Society,
Vol. 191,
Issue. 0,
p.
61.
Morris, Sidney A.
Ordman, Edward T.
and
Thompson, H. B.
1974.
Proceedings of the Second International Conference on the Theory of Groups.
Vol. 372,
Issue. ,
p.
504.
Morris, Sidney A.
Ordman, Edward T.
and
Thompson, H. B.
1974.
Proceedings of the Second International Conference on The Theory of Groups.
Vol. 372,
Issue. ,
p.
504.
Morris, Sidney A.
1975.
Free products of topological groups: Corrigendum.
Bulletin of the Australian Mathematical Society,
Vol. 12,
Issue. 3,
p.
480.
Brown, Ronald
and
Hardy, J. P. L.
1976.
Topological groupoids: I. universal constructions.
Mathematische Nachrichten,
Vol. 71,
Issue. 1,
p.
273.
Thomas, Barbara V. Smith
1977.
CATEGORIES OF TOPOLOGICAL GROUPS.
Quaestiones Mathematicae,
Vol. 2,
Issue. 1-3,
p.
355.
Fay, Temple H.
and
Smith-Thomas, Barbara V.
1979.
Remarks on the free product of two Hausdorff groups.
Archiv der Mathematik,
Vol. 33,
Issue. 1,
p.
57.
Mukhin, Yu. N.
1985.
Topological groups.
Journal of Soviet Mathematics,
Vol. 28,
Issue. 6,
p.
825.
Pyrch, N. M.
2012.
On Z-quotient mappings and homomorphisms of paratopological groups.
Journal of Mathematical Sciences,
Vol. 185,
Issue. 6,
p.
800.
İkeda, Kâzım İlhan
2013.
On the non-abelian global class field theory.
Annales mathématiques du Québec,
Vol. 37,
Issue. 2,
p.
129.
Vinhage, Kurt
2015.
On the rigidity of Weyl chamber flows and Schur multipliers as topological groups.
Journal of Modern Dynamics,
Vol. 9,
Issue. 01,
p.
25.
İkeda, Kâzım İlhan
2015.
Mathematics in the 21st Century.
Vol. 98,
Issue. ,
p.
45.
Vinhage, Kurt
and
Wang, Zhenqi Jenny
2019.
Local rigidity of higher rank homogeneous abelian actions: a complete solution via the geometric method.
Geometriae Dedicata,
Vol. 200,
Issue. 1,
p.
385.
Bandyopadhyay, Choiti
2021.
Free product on semihypergroups.
Semigroup Forum,
Vol. 102,
Issue. 1,
p.
28.
 Gα of a set {Gα} of topological groups is introduced. It is shown that it always exists, is unique and is algebraically isomorphic to the usual free product of the underlying groups. Further if each Gα is Hausdorff, then
Gα of a set {Gα} of topological groups is introduced. It is shown that it always exists, is unique and is algebraically isomorphic to the usual free product of the underlying groups. Further if each Gα is Hausdorff, then  Gα is Hausdorff and each Gα is a closed subgroup. Also
Gα is Hausdorff and each Gα is a closed subgroup. Also  Gα is a free topological group (respectively, maximally almost periodic) if each Gα is. This notion is then combined with the theory of varieties of topological groups developed by the author. For
Gα is a free topological group (respectively, maximally almost periodic) if each Gα is. This notion is then combined with the theory of varieties of topological groups developed by the author. For 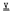 a variety of topological groups, the
a variety of topological groups, the 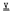 -product of groups in
-product of groups in 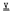 is defined. It is shown that the
is defined. It is shown that the 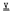 -product,
-product, 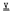
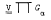 Gα of any set {Gα} of groups in
Gα of any set {Gα} of groups in 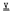 exists, is unique and is algebraically isomorphic to the usual varietal product. It is noted that the
exists, is unique and is algebraically isomorphic to the usual varietal product. It is noted that the 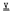 -product of Hausdorff groups is not necessarily Hausdorff, but is if
-product of Hausdorff groups is not necessarily Hausdorff, but is if 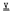 is abelian. Each Gα is a quotient group of
is abelian. Each Gα is a quotient group of 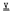
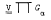 Gα. It is proved that the
Gα. It is proved that the 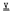 -product of free topological groups of
-product of free topological groups of 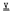 and projective topological groups of
and projective topological groups of 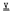 are of the same type. Finally it is shown that
are of the same type. Finally it is shown that 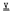
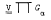 Gα is connected if and only if each Gα is connected.
Gα is connected if and only if each Gα is connected.