Article contents
THE FIXED POINT PROPERTY IN DIRECT SUMS AND MODULUS  $R(a, X)$
$R(a, X)$
Published online by Cambridge University Press: 28 June 2013
Abstract
We show that the direct sum 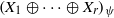 $\mathop{({X}_{1} \oplus \cdots \oplus {X}_{r} )}\nolimits_{\psi } $ with a strictly monotone norm has the weak fixed point property for nonexpansive mappings whenever
$\mathop{({X}_{1} \oplus \cdots \oplus {X}_{r} )}\nolimits_{\psi } $ with a strictly monotone norm has the weak fixed point property for nonexpansive mappings whenever 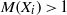 $M({X}_{i} )\gt 1$ for each
$M({X}_{i} )\gt 1$ for each 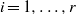 $i= 1, \ldots , r$. In particular,
$i= 1, \ldots , r$. In particular,  $\mathop{({X}_{1} \oplus \cdots \oplus {X}_{r} )}\nolimits_{\psi } $ enjoys the fixed point property if Banach spaces
$\mathop{({X}_{1} \oplus \cdots \oplus {X}_{r} )}\nolimits_{\psi } $ enjoys the fixed point property if Banach spaces 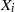 ${X}_{i} $ are uniformly nonsquare. This combined with the earlier results gives a definitive answer for
${X}_{i} $ are uniformly nonsquare. This combined with the earlier results gives a definitive answer for 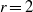 $r= 2$: a direct sum
$r= 2$: a direct sum  ${X}_{1} {\mathop{\oplus }\nolimits}_{\psi } {X}_{2} $ of uniformly nonsquare spaces with any monotone norm has the fixed point property. Our results are extended to asymptotically nonexpansive mappings in the intermediate sense.
${X}_{1} {\mathop{\oplus }\nolimits}_{\psi } {X}_{2} $ of uniformly nonsquare spaces with any monotone norm has the fixed point property. Our results are extended to asymptotically nonexpansive mappings in the intermediate sense.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright ©2013 Australian Mathematical Publishing Association Inc.
References
- 1
- Cited by

