 $\unicode[STIX]{x1D70B}$-DECOMPOSABILITY
$\unicode[STIX]{x1D70B}$-DECOMPOSABILITYPublished online by Cambridge University Press: 31 January 2018
We derive some structural properties of a trifactorised finite group 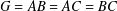 $G=AB=AC=BC$, where
$G=AB=AC=BC$, where 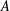 $A$,
$A$, 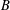 $B$, and
$B$, and 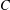 $C$ are subgroups of
$C$ are subgroups of 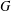 $G$, provided that
$G$, provided that 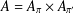 $A=A_{\unicode[STIX]{x1D70B}}\times A_{\unicode[STIX]{x1D70B}^{\prime }}$ and
$A=A_{\unicode[STIX]{x1D70B}}\times A_{\unicode[STIX]{x1D70B}^{\prime }}$ and  $B=B_{\unicode[STIX]{x1D70B}}\times B_{\unicode[STIX]{x1D70B}^{\prime }}$ are
$B=B_{\unicode[STIX]{x1D70B}}\times B_{\unicode[STIX]{x1D70B}^{\prime }}$ are  $\unicode[STIX]{x1D70B}$-decomposable groups, for a set of primes
$\unicode[STIX]{x1D70B}$-decomposable groups, for a set of primes  $\unicode[STIX]{x1D70B}$.
$\unicode[STIX]{x1D70B}$.
The first author was supported by Project VIP-008 of Yaroslavl P. Demidov State University and by a research grant from the Universitat de València as research visitor (Programa Propi d’Ajudes a la Investigació de la Universitat de València, Subprograma d’Atracció de Talent de VLC-Campus, Estades d’investigadors convidats (2017)). The second and third authors were supported by Proyecto MTM2014-54707-C3-1-P, Ministerio de Economía y Competitividad, Spain.