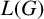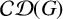Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Burrell, David
Cocke, William
and
McCulloch, Ryan
2024.
On Groups with Few Subgroups not in the Chermak–Delgado Lattice.
Arnold Mathematical Journal,
Vol. 10,
Issue. 2,
p.
265.
Cocke, William
and
McCulloch, Ryan
2024.
Central products and the Chermak–Delgado lattice.
Journal of Pure and Applied Algebra,
Vol. 228,
Issue. 12,
p.
107769.
Liu, Guojie
and
Song, Qiangwei
2025.
Finite groups with at most six subgroups not in the Chermak-Delgado lattice.
Electronic Research Archive,
Vol. 33,
Issue. 9,
p.
5769.