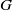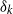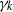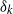Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Acciarri, Cristina
and
Shumyatsky, Pavel
2014.
On finite groups in which coprime commutators are covered by few cyclic subgroups.
Journal of Algebra,
Vol. 407,
Issue. ,
p.
358.
DE ANDRADE, AGENOR FREITAS
and
SHUMYATSKY, PAVEL
2016.
BOUNDING THE ORDER OF THE NILPOTENT RESIDUAL OF A FINITE GROUP.
Bulletin of the Australian Mathematical Society,
Vol. 94,
Issue. 2,
p.
273.
Freitas de Andrade, Agenor
and
Carrazedo Dantas, Alex
2018.
A sufficient condition for nilpotency of the nilpotent residual of a finite group.
Journal of Group Theory,
Vol. 21,
Issue. 2,
p.
289.
de Melo, Emerson
Lima, Aline de Souza
and
Shumyatsky, Pavel
2018.
Nilpotent residual of fixed points.
Archiv der Mathematik,
Vol. 111,
Issue. 1,
p.
13.
Acciarri, Cristina
and
Shumyatsky, Pavel
2019.
On groups in which Engel sinks are cyclic.
Journal of Algebra,
Vol. 539,
Issue. ,
p.
366.
de Melo, Emerson
2019.
Nilpotent residual and fitting subgroup of fixed points in finite groups.
Journal of Group Theory,
Vol. 22,
Issue. 6,
p.
1059.
DE MELO, EMERSON
and
SHUMYATSKY, PAVEL
2019.
FITTING SUBGROUP AND NILPOTENT RESIDUAL OF FIXED POINTS.
Bulletin of the Australian Mathematical Society,
Vol. 100,
Issue. 1,
p.
61.
Bastos, Raimundo
and
Monetta, Carmine
2019.
Coprime commutators in finite groups.
Communications in Algebra,
Vol. 47,
Issue. 10,
p.
4137.
Acciarri, Cristina
Shumyatsky, Pavel
and
Silveira, Danilo
2019.
Engel sinks of fixed points in finite groups.
Journal of Pure and Applied Algebra,
Vol. 223,
Issue. 11,
p.
4592.
Detomi, Eloisa
Morigi, Marta
and
Shumyatsky, Pavel
2021.
Strong conciseness of coprime and anti-coprime commutators.
Annali di Matematica Pura ed Applicata (1923 -),
Vol. 200,
Issue. 3,
p.
945.
Shumyatsky, Pavel
2022.
Finite-by-Nilpotent Groups and a Variation of the BFC-Theorem.
Mediterranean Journal of Mathematics,
Vol. 19,
Issue. 5,
de las Heras, Iker
Pintonello, Matteo
and
Shumyatsky, Pavel
2023.
Strong conciseness of coprime commutators in profinite groups.
Journal of Algebra,
Vol. 633,
Issue. ,
p.
1.
Rodrigues, Eliana
de Melo, Emerson
and
Ercan, Gülin
2024.
Nilpotent residual of a finite group.
Journal of Algebra,
Vol. 641,
Issue. ,
p.
534.
Detomi, Eloisa
Morigi, Marta
and
Shumyatsky, Pavel
2025.
Commuting probability for the Sylow subgroups of a profinite group.
Mathematische Zeitschrift,
Vol. 309,
Issue. 3,