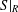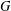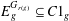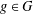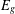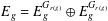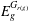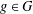Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Paques, Antonio
2018.
Advances in Mathematics and Applications.
p.
247.
Ávila, Jesús
Marín, Víctor
and
Pinedo, Héctor
2020.
Isomorphism Theorems for Groupoids and Some Applications.
International Journal of Mathematics and Mathematical Sciences,
Vol. 2020,
Issue. ,
p.
1.
Marín, Víctor
and
Pinedo, Héctor
2020.
Partial groupoid actions on R-categories: Globalization and the smash product.
Journal of Algebra and Its Applications,
Vol. 19,
Issue. 05,
p.
2050083.
Paques, Antonio
and
Tamusiunas, Thaísa
2021.
On the Galois map for groupoid actions.
Communications in Algebra,
Vol. 49,
Issue. 3,
p.
1037.
Lautenschlaeger, Wesley G.
and
Tamusiunas, Thaísa
2023.
Maximal Ordered Groupoids and a Galois Correspondence for Inverse Semigroup Orthogonal Actions.
Applied Categorical Structures,
Vol. 31,
Issue. 5,
Pedrotti, Juliana
and
Tamusiunas, Thaísa
2023.
Injectivity of the Galois Map.
Bulletin of the Brazilian Mathematical Society, New Series,
Vol. 54,
Issue. 1,
Garcia, Christian
and
Tamusiunas, Thaísa
2024.
A Galois correspondence for K-rings.
Journal of Algebra,
Vol. 640,
Issue. ,
p.
74.
Lautenschlaeger, Wesley G.
and
Tamusiunas, Thaísa
2025.
Galois correspondence for partial groupoid actions.
Communications in Algebra,
Vol. 53,
Issue. 9,
p.
3778.