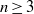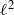Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Bargetz, Christian
Reich, Simeon
and
Zalas, Rafał
2018.
Convergence properties of dynamic string-averaging projection methods in the presence of perturbations.
Numerical Algorithms,
Vol. 77,
Issue. 1,
p.
185.
Cegielski, Andrzej
Reich, Simeon
and
Zalas, Rafał
2018.
Regular Sequences of Quasi-Nonexpansive Operators and Their Applications.
SIAM Journal on Optimization,
Vol. 28,
Issue. 2,
p.
1508.
Bargetz, Christian
Kolobov, Victor I.
Reich, Simeon
and
Zalas, Rafał
2019.
Linear convergence rates for extrapolated fixed point algorithms.
Optimization,
Vol. 68,
Issue. 1,
p.
163.
Kopecká, Eva
2020.
When products of projections diverge.
Journal of the London Mathematical Society,
Vol. 102,
Issue. 1,
p.
345.