No CrossRef data available.
Published online by Cambridge University Press: 10 June 2020
For a positive real number  $t$, define the harmonic continued fraction We prove that
$t$, define the harmonic continued fraction We prove that 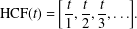 $$\begin{eqnarray}\text{HCF}(t)=\biggl[\frac{t}{1},\frac{t}{2},\frac{t}{3},\ldots \biggr].\end{eqnarray}$$
$$\begin{eqnarray}\text{HCF}(t)=\biggl[\frac{t}{1},\frac{t}{2},\frac{t}{3},\ldots \biggr].\end{eqnarray}$$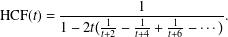 $$\begin{eqnarray}\text{HCF}(t)=\frac{1}{1-2t(\frac{1}{t+2}-\frac{1}{t+4}+\frac{1}{t+6}-\cdots \,)}.\end{eqnarray}$$
$$\begin{eqnarray}\text{HCF}(t)=\frac{1}{1-2t(\frac{1}{t+2}-\frac{1}{t+4}+\frac{1}{t+6}-\cdots \,)}.\end{eqnarray}$$