No CrossRef data available.
Article contents
EXISTENCE OF K-MULTIMAGIC SQUARES AND MAGIC SQUARES OF kth POWERS WITH DISTINCT ENTRIES
Published online by Cambridge University Press: 11 February 2025
Abstract
We demonstrate the existence of K-multimagic squares of order N consisting of 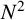 $N^2$ distinct integers whenever
$N^2$ distinct integers whenever 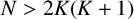 $N> 2K(K+1)$. This improves our earlier result [D. Flores, ‘A circle method approach to K-multimagic squares’, preprint (2024), arXiv:2406.08161] in which we only required
$N> 2K(K+1)$. This improves our earlier result [D. Flores, ‘A circle method approach to K-multimagic squares’, preprint (2024), arXiv:2406.08161] in which we only required 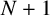 $N+1$ distinct integers. Additionally, we present a direct method by which our analysis of the magic square system may be used to show the existence of
$N+1$ distinct integers. Additionally, we present a direct method by which our analysis of the magic square system may be used to show the existence of  $N \times N$ magic squares consisting of distinct kth powers when
$N \times N$ magic squares consisting of distinct kth powers when 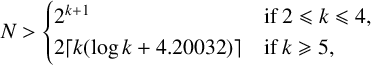 $$ \begin{align*}N> \begin{cases} 2^{k+1} & \text{if}\ 2 \leqslant k \leqslant 4, \\ 2 \lceil k(\log k + 4.20032) \rceil & \text{if}\ k \geqslant 5, \end{cases}\end{align*} $$
$$ \begin{align*}N> \begin{cases} 2^{k+1} & \text{if}\ 2 \leqslant k \leqslant 4, \\ 2 \lceil k(\log k + 4.20032) \rceil & \text{if}\ k \geqslant 5, \end{cases}\end{align*} $$
improving on a recent result by Rome and Yamagishi [‘On the existence of magic squares of powers’, preprint (2024), arxiv:2406.09364].
MSC classification
Information
- Type
- Research Article
- Information
- Copyright
- © The Author(s), 2025. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.
Footnotes
The author acknowledges the support of Purdue University, which provided funding for this research through the Ross–Lynn Research Scholar Fund.


