Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Smith, Richard L
1988.
Extreme value theory for dependent sequences via the stein-chen method of poisson approximation.
Stochastic Processes and their Applications,
Vol. 30,
Issue. 2,
p.
317.
Bacon, P.J.
Ball, Frank
and
Blackwell, Paul
1991.
A model for territory and group formation in a heterogeneous habitat.
Journal of Theoretical Biology,
Vol. 148,
Issue. 4,
p.
445.
Grycko, E.
1994.
Extreme values and tests for randomness.
Computers & Mathematics with Applications,
Vol. 28,
Issue. 7,
p.
47.
Hüsler, J.
and
Kratz, M.
1995.
Rate of Poisson approximation of the number of exceedances of nonstationary normal sequences.
Stochastic Processes and their Applications,
Vol. 55,
Issue. 2,
p.
301.
Hsing, Tailen
Hüsler, Jürg
and
Reiss, Rolf-Dieter
1996.
The extremes of a triangular array of normal random variables.
The Annals of Applied Probability,
Vol. 6,
Issue. 2,
Kratz, Marie F.
and
Rootzén, Holger
1997.
On the rate of convergence for extremes of mean square differentiable stationary normal processes.
Journal of Applied Probability,
Vol. 34,
Issue. 04,
p.
908.
Graça Temido, M.
2000.
Mixture results for extremal behaviour of strongly dependent nonstationary Gaussian sequences.
Test,
Vol. 9,
Issue. 2,
p.
439.
Nadarajah, Saralees
2003.
Stochastic Processes: Modelling and Simulation.
Vol. 21,
Issue. ,
p.
607.
Barbe, Ph.
and
McCormick, W.P.
2004.
Second-order expansion for the maximum of some stationary Gaussian sequences.
Stochastic Processes and their Applications,
Vol. 110,
Issue. 2,
p.
315.
Mladenovic, Pavle
and
Mladenovic, Pavle
2005.
A generalization of the Mejzler - De Haan theorem.
Теория вероятностей и ее применения,
Vol. 50,
Issue. 1,
p.
177.
Hannig, Jan
and
Lee, Thomas C. M
2006.
Robust SiZer for Exploration of Regression Structures and Outlier Detection.
Journal of Computational and Graphical Statistics,
Vol. 15,
Issue. 1,
p.
101.
Mladenovic, P.
2006.
A Generalization of the Mejzler--de Haan Theorem.
Theory of Probability & Its Applications,
Vol. 50,
Issue. 1,
p.
141.
Peng, Zuoxiang
Nadarajah, Saralees
and
Lin, Fuming
2010.
Convergence Rate of Extremes for the General Error Distribution.
Journal of Applied Probability,
Vol. 47,
Issue. 03,
p.
668.
Falk, Michael
Hüsler, Jürg
and
Reiss, Rolf-Dieter
2011.
Laws of Small Numbers: Extremes and Rare Events.
p.
357.
French, Joshua P.
and
Davis, Richard A.
2013.
The asymptotic distribution of the maxima of a Gaussian random field on a lattice.
Extremes,
Vol. 16,
Issue. 1,
p.
1.
Nadarajah, Saralees
2015.
Complete asymptotic expansions for normal extremes.
Statistics & Probability Letters,
Vol. 103,
Issue. ,
p.
127.
Kokoszka, Piotr
and
Xiong, Qian
2018.
Extremes of projections of functional time series on data–driven basis systems.
Extremes,
Vol. 21,
Issue. 2,
p.
177.
Ghosh, Santu
Ayyala, Deepak Nag
and
Hellebuyck, Rafael
2021.
Two-sample high dimensional mean test based on prepivots.
Computational Statistics & Data Analysis,
Vol. 163,
Issue. ,
p.
107284.
Zhu, Yijun
and
Xiao, Han
2023.
Robust and Multivariate Statistical Methods.
p.
451.
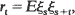 let
let 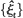 be independent and standard normal, and write
be independent and standard normal, and write 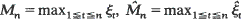 . In this paper we find bounds on
. In this paper we find bounds on 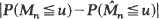 which are roughly of the order
which are roughly of the order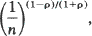 where ρ is the maximal correlation, ρ =sup {0, r 1 , r 2, …}. It is further shown that, at least for m-dependent sequences, the bounds are of the right order and, in a simple example, the errors are evaluated numerically. Bounds of the same order on the rate of convergence of the point processes of exceedances of one or several levels are obtained using a ‘representation' approach (which seems to be of rather wide applicability). As corollaries we obtain rates of convergence of several functionals of the point processes, including the joint distribution function of the k largest values amongst ξ1, …, ξn.
where ρ is the maximal correlation, ρ =sup {0, r 1 , r 2, …}. It is further shown that, at least for m-dependent sequences, the bounds are of the right order and, in a simple example, the errors are evaluated numerically. Bounds of the same order on the rate of convergence of the point processes of exceedances of one or several levels are obtained using a ‘representation' approach (which seems to be of rather wide applicability). As corollaries we obtain rates of convergence of several functionals of the point processes, including the joint distribution function of the k largest values amongst ξ1, …, ξn.
