Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Antonelli, Peter L.
and
Morgan, Kenneth
1978.
On the persistence of random genetic drift.
Journal of Applied Probability,
Vol. 15,
Issue. 01,
p.
179.
Antonelli, Peter L.
1978.
The geometry of random drift IV. Random time substitutions and stationary densities.
Advances in Applied Probability,
Vol. 10,
Issue. 03,
p.
563.
Antonelli, Peter L.
1978.
An asymptotic formula for the transition density of random genetic drift.
Journal of Applied Probability,
Vol. 15,
Issue. 01,
p.
184.
Antonelli, Peter L.
1979.
The geometry of random drift V. Axiomatic derivation of the WFK diffusion from a variational principle.
Advances in Applied Probability,
Vol. 11,
Issue. 03,
p.
502.
Antonelli, Peter L.
Chapin, Jared
and
Voorhees, B. H.
1980.
The geometry of random genetic drift VI. A random selection diffusion model.
Advances in Applied Probability,
Vol. 12,
Issue. 01,
p.
50.
Jorde, Lynn B.
1980.
Current Developments in Anthropological Genetics.
p.
135.
Barton, N H
1986.
The effects of linkage and density-dependent regulation on gene flow.
Heredity,
Vol. 57,
Issue. 3,
p.
415.
Antonelli, P.L
Elliott, R.J
and
Seymour, R.M
1987.
Nonlinear filtering and Riemannian scalar curvature, R.
Advances in Applied Mathematics,
Vol. 8,
Issue. 3,
p.
237.
Barton, N. H.
and
Turelli, Michael
1987.
Adaptive landscapes, genetic distance and the evolution of quantitative characters.
Genetical Research,
Vol. 49,
Issue. 2,
p.
157.
Akin, E.
1987.
Dynamical Systems.
Vol. 287,
Issue. ,
p.
139.
Barton, N.H.
and
Rouhani, S.
1987.
The frequency of shifts between alternative equilibria.
Journal of Theoretical Biology,
Vol. 125,
Issue. 4,
p.
397.
Antonelli, P.
Bradbury, R.
Buck, R.
Reichelt, R.
and
Elliott, R.
1988.
Nonlinear prediction of crown–of–thorns outbreaks on the great barrier reef.
Stochastic Analysis and Applications,
Vol. 6,
Issue. 4,
p.
349.
Antonelli, P.
Bradbury, R.
Buck, R.
Reichelt, R.
and
Elliott, R.
1988.
Acanthaster and the Coral Reef: A Theoretical Perspective.
Vol. 88,
Issue. ,
p.
314.
Hines, W.G.S.
1990.
A report on evolutionarily stable strategy: Modelling the effects of behavior on evolution.
Mathematical and Computer Modelling,
Vol. 14,
Issue. ,
p.
694.
Akin, Ethan
1990.
Mathematical and Statistical Developments of Evolutionary Theory.
p.
1.
Antonelli, P. L.
Bradbury, R. H.
and
Reichelt, R. E.
1993.
Multiple time-scale diffusion models of starfish and coral state changes over the whole great barrier reef.
Open Systems & Information Dynamics,
Vol. 2,
Issue. 1,
p.
95.
Hines, W. G. S.
1994.
ESS modelling of diploid populations II: stability analysis of possible equilibria.
Advances in Applied Probability,
Vol. 26,
Issue. 2,
p.
361.
Hines, W. G. S.
1995.
New Trends in Dynamic Games and Applications.
p.
467.
Baake, Ellen
and
von Haeseler, Arndt
1999.
Distance Measures in Terms of Substitution Processes.
Theoretical Population Biology,
Vol. 55,
Issue. 2,
p.
166.
Khatri, Bhavin S.
2016.
Quantifying evolutionary dynamics from variant-frequency time series.
Scientific Reports,
Vol. 6,
Issue. 1,
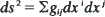 where (gij ) is the inverse of the covariance matrix of the diffusion equation. This permits the study of the geometry associated with a diffusion equation, since the matrix (gij ) is the fundamental tensor of the Riemannian space (X, gij ), and of a diffusion process in terms of Brownian motion. For the diffusion equation approximation to random drift with n alleles the covariance matrix is that of a multinomial distribution. The resulting stochastic distance is equal to twice the genetic distance as defined by Cavalli-Sforza and Edwards and is a generalization of the angular transformation of Fisher to n alleles. The geometry associated with the diffusion equation for random drift with n alleles is that of a
where (gij ) is the inverse of the covariance matrix of the diffusion equation. This permits the study of the geometry associated with a diffusion equation, since the matrix (gij ) is the fundamental tensor of the Riemannian space (X, gij ), and of a diffusion process in terms of Brownian motion. For the diffusion equation approximation to random drift with n alleles the covariance matrix is that of a multinomial distribution. The resulting stochastic distance is equal to twice the genetic distance as defined by Cavalli-Sforza and Edwards and is a generalization of the angular transformation of Fisher to n alleles. The geometry associated with the diffusion equation for random drift with n alleles is that of a  part of an (n − 1)-sphere of radius two. We also show that the diffusion equation for random drift is not spherical Brownian motion, although it is approximated by it near the centroid of frequency space.
part of an (n − 1)-sphere of radius two. We also show that the diffusion equation for random drift is not spherical Brownian motion, although it is approximated by it near the centroid of frequency space.
