Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Shanthikumar, J.G.
and
Sumita, U.
1984.
A central limit theorem for random sums of random variables.
Operations Research Letters,
Vol. 3,
Issue. 3,
p.
153.
Shanthikumar, J. G.
1984.
Processes with new better than used first-passage times.
Advances in Applied Probability,
Vol. 16,
Issue. 03,
p.
667.
Jensen, Uwe
1985.
DGOR.
Vol. 1984,
Issue. ,
p.
548.
Sumita, Ushio
and
Shanthikumar, J. George
1985.
A class of correlated cumulative shock models.
Advances in Applied Probability,
Vol. 17,
Issue. 02,
p.
347.
Rangan, A.
and
Grace, R.Esther
1988.
On a class of optimal preventive replacement policies of systems subject to shocks.
Microelectronics Reliability,
Vol. 28,
Issue. 4,
p.
563.
Sumita, Ushio
and
Shanthikumar, J. George
1988.
An age-dependent counting process generated from a renewal process.
Advances in Applied Probability,
Vol. 20,
Issue. 04,
p.
739.
Kováts, Antal
and
Móri, Tamás F.
1992.
Ageing properties of certain dependent geometric sums.
Journal of Applied Probability,
Vol. 29,
Issue. 03,
p.
655.
Kováts, A.
and
Móri, T.
1992.
Probability Theory and Applications.
p.
125.
LI, ZEHUI
CHAN, LING-YAU
and
YUAN, ZHIXIN
1999.
FAILURE TIME DISTRIBUTION UNDER A δ-SHOCK MODEL AND ITS APPLICATION TO ECONOMIC DESIGN OF SYSTEMS.
International Journal of Reliability, Quality and Safety Engineering,
Vol. 06,
Issue. 03,
p.
237.
Kijima, Masaaki
Li, Haijun
and
Shaked, Moshe
2001.
Stochastic Processes: Theory and Methods.
Vol. 19,
Issue. ,
p.
471.
Mallor, Fermín
and
Santos, Javier
2003.
Reliability of systems subject to shocks with a stochastic dependence for the damages.
Test,
Vol. 12,
Issue. 2,
p.
427.
Shaked, M.
and
Li, H.
2004.
Encyclopedia of Statistical Sciences.
Lam, Yeh
and
Zhang, Yuan Lin
2004.
A shock model for the maintenance problem of a repairable system.
Computers & Operations Research,
Vol. 31,
Issue. 11,
p.
1807.
Shaked, M.
and
Li, H.
2005.
Encyclopedia of Statistical Sciences.
Tang, Ya-yong
and
Lam, Yeh
2006.
A δ-shock maintenance model for a deteriorating system.
European Journal of Operational Research,
Vol. 168,
Issue. 2,
p.
541.
Bai, J.-M.
Li, Z.-H.
and
Kong, X.-B.
2006.
Generalized Shock Models Based on a Cluster Point Process.
IEEE Transactions on Reliability,
Vol. 55,
Issue. 3,
p.
542.
Chen, Jinyuan
and
Li, Zehui
2008.
An extended extreme shock maintenance model for a deteriorating system.
Reliability Engineering & System Safety,
Vol. 93,
Issue. 8,
p.
1123.
Finkelstein, Maxim
2008.
Encyclopedia of Quantitative Risk Analysis and Assessment.
Yeh Lam
2009.
A Geometric Process $\delta$-Shock Maintenance Model.
IEEE Transactions on Reliability,
Vol. 58,
Issue. 2,
p.
389.
Ma, Ming
and
Li, Zehui
2010.
Life behavior of censored δ-shock model.
Indian Journal of Pure and Applied Mathematics,
Vol. 41,
Issue. 2,
p.
401.

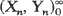 . Two models, depending on whether the magnitude of the nth shock
. Two models, depending on whether the magnitude of the nth shock 