No CrossRef data available.
Published online by Cambridge University Press: 01 July 2016
Let 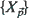 be a real-valued, homogeneous, and isotropic random field indexed in
be a real-valued, homogeneous, and isotropic random field indexed in 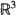 . When restricted to those indices
. When restricted to those indices 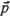 with
with  , the Euclidean length of
, the Euclidean length of 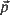 , equal to r (a positive constant), then the random field
, equal to r (a positive constant), then the random field 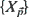 resides on the surface of a sphere of radius r. Using a modified stratified spherical sampling plan (Brown (1993))
resides on the surface of a sphere of radius r. Using a modified stratified spherical sampling plan (Brown (1993))  on the sphere, define
on the sphere, define 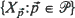 to be a realization of the random process and
to be a realization of the random process and 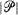 to be the cardinality of
to be the cardinality of  . Without specifying the dependence structure of
. Without specifying the dependence structure of  nor the marginal distribution of the
nor the marginal distribution of the 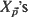 , conditions for asymptotic normality of the standardized sample mean,
, conditions for asymptotic normality of the standardized sample mean, 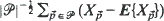 , are given. The conditions on
, are given. The conditions on 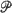 and
and  are motivated by the ideas and results for dependent stationary sequences.
are motivated by the ideas and results for dependent stationary sequences.
This research was partially supported by NSF grant DMS-94.04130.